RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities

RD Sharma Solutions for Class 10 Maths Chapter 6 Exercise 6.1 Page No: 6.43

Prove the following trigonometric identities:

1. (1 – cos2 A) cosec2 A = 1

Solution:

Taking the L.H.S,

(1 – cos2 A) cosec2 A

= (sin2 A) cosec2 A [∵ sin2 A + cos2 A = 1 ⇒1 – sin2 A = cos2 A]

= 12

= 1 = R.H.S

– Hence Proved

2. (1 + cot2 A) sin2 A = 1 

Solution: 

By using the identity,

cosec2 A – cot2 A = 1 ⇒ cosec2 A = cot2 A + 1

Taking,

L.H.S = (1 + cot2 A) sin2 A

= cosec2 A sin2 A

= (cosec A sin A)2

= ((1/sin A) × sin A)2

= (1)2

= 1

= R.H.S

– Hence Proved

3. tan2 θ cos2 θ = 1 − cos2 θ 

Solution: 

We know that,

sin2 θ + cos2 θ = 1

Taking,

L.H.S = tan2 θ cos2 θ

= (tan θ × cos θ)2

= (sin θ)2

= sin2 θ

= 1 – cos2 θ

= R.H.S

– Hence Proved

4. cosec θ √(1 – cos2 θ) = 1

Solution:

Using identity,

sin2 θ + cos2 θ = 1  ⇒ sin2 θ = 1 – cos2 θ

Taking L.H.S,

L.H.S = cosec θ √(1 – cos2 θ)

= cosec θ √( sin2 θ)

= cosec θ x sin θ

= 1

= R.H.S

– Hence Proved

5. (sec2 θ − 1)(cosec2 θ − 1) = 1 

Solution:

Using identities,

(sec2 θ − tan2 θ) = 1 and (cosec2 θ − cot2 θ) = 1

We have,

L.H.S = (sec2 θ – 1)(cosec2θ – 1)

= tan2θ × cot2θ

= (tan θ × cot θ)2

= (tan θ × 1/tan θ)2

= 12

= 1

= R.H.S

– Hence Proved

6. tan θ + 1/ tan θ = sec θ cosec θ

Solution:

We have,

L.H.S = tan θ + 1/ tan θ

= (tan2 θ + 1)/ tan θ

= sec2 θ / tan θ [∵ sec2 θ − tan2 θ = 1]

= (1/cos2 θ) x 1/ (sin θ/cos θ) [∵ tan θ = sin θ / cos θ]

= cos θ/ (sin θ x cos2 θ)

= 1/ cos θ x 1/ sin θ

= sec θ x cosec θ

= sec θ cosec θ

= R.H.S

– Hence Proved

7. cos θ/ (1 – sin θ) = (1 + sin θ)/ cos θ

Solution:

We know that,

sin2 θ + cos2 θ = 1

So, by multiplying both the numerator and the denominator by (1+ sin θ), we get

L.H.S =

= R.H.S

– Hence Proved

8. cos θ/ (1 + sin θ) = (1 – sin θ)/ cos θ

Solution:

We know that,

sin2 θ + cos2 θ = 1

So, by multiplying both the numerator and the denominator by (1- sin θ), we get

L.H.S =

= R.H.S

– Hence Proved

9. cos2 θ + 1/(1 + cot2 θ) = 1

Solution:

We already know that,

cosec2 θ − cot2 θ = 1 and sin2 θ + cos2 θ = 1

Taking L.H.S,

= cos2 A + sin2 A

= 1

= R.H.S

– Hence Proved

10. sin2 A + 1/(1 + tan 2 A) = 1

Solution:

We already know that,

sec2 θ − tan2 θ = 1 and sin2 θ + cos2 θ = 1

Taking L.H.S,

= sin2 A + cos2 A

= 1

= R.H.S

– Hence Proved

11.

Solution:

We know that, sin2 θ + cos2 θ = 1

Taking the L.H.S,

= cosec θ – cot θ

= R.H.S

– Hence Proved

12. 1 – cos θ/ sin θ = sin θ/ 1 + cos θ

Solution:

We know that,

sin2 θ + cos2 θ = 1

So, by multiplying both the numerator and the denominator by (1+ cos θ), we get

= R.H.S

– Hence Proved

13. sin θ/ (1 – cos θ) = cosec θ + cot θ

Solution:

 

Taking L.H.S,

= cosec θ + cot θ

= R.H.S

– Hence Proved

14. (1 – sin θ) / (1 + sin θ) = (sec θ – tan θ)2

Solution:

Taking the L.H.S,

= (sec θ – tan θ)2

= R.H.S

– Hence Proved

15. 

Solution:

Taking L.H.S,

= cot θ

= R.H.S

– Hence Proved

16. tan2 θ − sin2 θ = tan2 θ sin2 θ 

Solution:

Taking L.H.S,

L.H.S = tan2 θ − sin2 θ 

= tan2 θ sin2 θ

= R.H.S

– Hence Proved

17. (cosec θ + sin θ)(cosec θ – sin θ) = cot2θ + cos2θ 

Solution:

Taking L.H.S = (cosec θ + sin θ)(cosec θ – sin θ)

On multiplying we get,

= cosec2 θ – sin2 θ

= (1 + cot2 θ) – (1 – cos2 θ) [Using cosec2 θ − cot2 θ = 1 and sin2 θ + cos2 θ = 1]

= 1 + cot2 θ – 1 + cos2 θ

= cot2 θ + cos2 θ

= R.H.S

– Hence Proved

18. (sec θ + cos θ) (sec θ – cos θ) = tan2 θ + sin2 θ 

Solution:

Taking L.H.S = (sec θ + cos θ)(sec θ – cos θ)

On multiplying we get,

= sec2 θ – sin2 θ

= (1 + tan2 θ) – (1 – sin2 θ) [Using sec2 θ − tan2 θ = 1 and sin2 θ + cos2 θ = 1]

= 1 + tan2 θ – 1 + sin2 θ

= tan 2 θ + sin 2 θ

= R.H.S

– Hence Proved

19. sec A(1- sin A) (sec A + tan A) = 1

Solution:

Taking L.H.S = sec A(1 – sin A)(sec A + tan A)

Substituting sec A = 1/cos A and tan A =sin A/cos A in the above we have,

L.H.S = 1/cos A (1 – sin A)(1/cos A + sin A/cos A)

= 1 – sin2 A / cos2 A [After taking L.C.M]

= cos2 A / cos2 A [∵ 1 – sin2 A = cos2 A]

= 1

= R.H.S

– Hence Proved

20. (cosec A – sin A)(sec A – cos A)(tan A + cot A) = 1 

Solution:

Taking L.H.S = (cosec A – sin A)(sec A – cos A)(tan A + cot A)

= (cos2 A/ sin A) (sin2 A/ cos A) (1/ sin A cos A) [∵ sin2 θ + cos2 θ = 1]

= (sin A  cos A)  (1/ cos A sin A)

= 1

= R.H.S

– Hence Proved

21. (1 + tan2 θ)(1 – sin θ)(1 + sin θ) = 1

Solution:

Taking L.H.S = (1 + tan2θ)(1 – sin θ)(1 + sin θ)

And, we know sin2 θ + cos2 θ = 1 and sec2 θ – tan2 θ = 1

So,

L.H.S = (1 + tan2 θ)(1 – sin θ)(1 + sin θ)

= (1 + tan2 θ){(1 – sin θ)(1 + sin θ)}

= (1 + tan2 θ)(1 – sin2 θ)

= sec2 θ (cos2 θ)

= (1/ cos2 θ) x cos2 θ

= 1

= R.H.S

– Hence Proved

22. sin2 A cot2 A + cos2 A tan2 A = 1

Solution:

We know that,

cot2 A = cos2 A/ sin2 A and tan2 A = sin2 A/cos2 A

Substituting the above in L.H.S, we get

L.H.S = sin2 A cot2 A + cos2 A tan2 A

= {sin2 A (cos2 A/ sin2 A)} + {cos2 A (sin2 A/cos2 A)}

= cos2 A + sin2 A

= 1 [∵ sin2 θ + cos2 θ = 1]

= R.H.S

– Hence Proved

23.

Solution:

(i) Taking the L.H.S and using sin2 θ + cos2 θ = 1, we have

L.H.S = cot θ – tan θ

= R.H.S

– Hence Proved

(ii) Taking the L.H.S and using sin2 θ + cos2 θ = 1, we have

L.H.S = tan θ – cot θ

= R.H.S

– Hence Proved

24. (cos2 θ/ sin θ) – cosec θ + sin θ = 0

Solution:

Taking L.H.S and using sin2 θ + cos2 θ = 1, we have

= – sin θ + sin θ

= 0

= R.H.S

• Hence proved

25.

Solution:

Taking L.H.S,

= 2 sec2 A

= R.H.S

• Hence proved

26. 

Solution:

Taking the LHS and using sin2 θ + cos2 θ = 1, we have

= 2/ cos θ

= 2 sec θ

= R.H.S

• Hence proved

27.

Solution:

 

Taking the LHS and using sin2 θ + cos2 θ = 1, we have

= R.H.S

• Hence proved

28.

Solution:

Taking L.H.S,

Using sec2 θ − tan2 θ = 1 and cosec2 θ − cot2 θ = 1

= R.H.S

• Hence proved

29.

Solution:

Taking L.H.S and using sin2 θ + cos2 θ = 1, we have

= R.H.S

• Hence proved

30.

Solution:

Taking LHS, we have

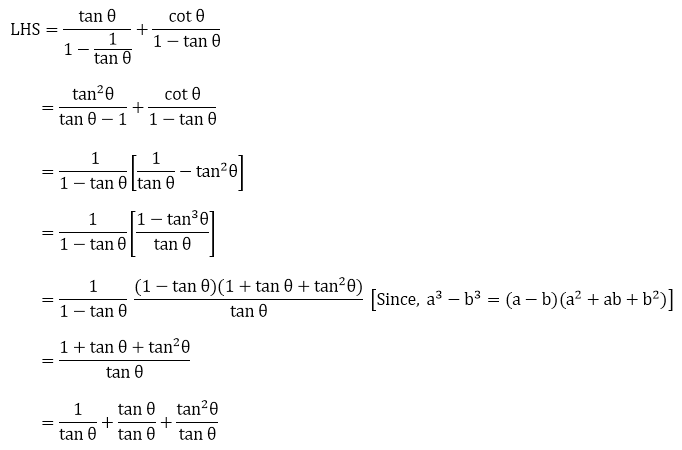
= 1 + tan θ + cot θ

= R.H.S

• Hence proved

31. sec6 θ = tan6 θ + 3 tan2 θ sec2 θ + 1

Solution: 

From trig. Identities we have,

sec2 θ − tan2 θ = 1

On cubing both sides,

(sec2θ − tan2θ)3 = 1

sec6 θ − tan6 θ − 3sec2 θ tan2 θ(sec2 θ − tan2 θ) = 1

[Since, (a – b)3 = a3 – b3 – 3ab(a – b)]

sec6 θ − tan6 θ − 3sec2 θ tan2 θ = 1

⇒ sec6 θ = tan6 θ + 3sec2 θ tan2 θ + 1

Hence, L.H.S = R.H.S

• Hence proved

32. cosec6 θ = cot6 θ + 3cot2 θ cosec2 θ + 1

Solution:

From trig. Identities we have,

cosec2 θ − cot2 θ = 1

On cubing both sides,

(cosec2 θ − cot2 θ)3 = 1

cosec6 θ − cot6 θ − 3cosec2 θ cot2 θ (cosec2 θ − cot2 θ) = 1

[Since, (a – b)3 = a3 – b3 – 3ab(a – b)]

cosec6 θ − cot6 θ − 3cosec2 θ cot2 θ = 1

⇒ cosec6 θ = cot6 θ + 3 cosec2 θ cot2 θ + 1

Hence, L.H.S = R.H.S

• Hence proved

33.

Solution:

Taking L.H.S and using sec2 θ − tan2 θ = 1 ⇒ 1 + tan2 θ = sec2 θ

= R.H.S

• Hence proved

34.

Solution:

Taking L.H.S and using the identity sin2A + cos2A = 1, we get

sin2A = 1 − cos2A

⇒ sin2A = (1 – cos A)(1 + cos A)

• Hence proved

35.

Solution:

We have,

Rationalizing the denominator and numerator with (sec A + tan A) and using sec2 θ − tan2 θ = 1 we get,

= R.H.S

• Hence proved

36.

Solution:

We have,

On multiplying numerator and denominator by (1 – cos A), we get

= R.H.S

• Hence proved

37. (i)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with √(1 + sin A), we get

= R.H.S

• Hence proved

(ii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= 2 cosec A

= R.H.S

• Hence proved

38. Prove that:

(i)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= 2 cosec θ

= R.H.S

• Hence proved

(ii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= R.H.S

• Hence proved

(iii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

 

= 2 cosec θ

= R.H.S

• Hence proved

(iv)

Solution:

Taking L.H.S, we have

= R.H.S

• Hence proved

39.

Solution:

 

Taking LHS = (sec A – tan A)2 , we have

= R.H.S

• Hence proved

40.

Solution:

Taking L.H.S and rationalizing the numerator and denominator with (1 – cos A), we get

= (cosec A – cot A)2

= (cot A – cosec A)2

= R.H.S

• Hence proved

41.

Solution:

Considering L.H.S and taking L.C.M and on simplifying we have,

= 2 cosec A cot A = RHS

• Hence proved

42.

Solution:

Taking LHS, we have

= cos A + sin A

= RHS

• Hence proved

43.

Solution:

Considering L.H.S and taking L.C.M and on simplifying we have,

= 2 sec2 A

= RHS

• Hence proved

RD Sharma Solutions for Class 10 Maths Chapter 6 Exercise 6.2 Page No: 6.54

1. If cos θ = 4/5, find all other trigonometric ratios of angle θ. 

Solution:

 

We have,

cos θ = 4/5

And we know that,

sin θ = √(1 – cos2 θ)

⇒ sin θ = √(1 – (4/5)2)

= √(1 – (16/25))

= √[(25 – 16)/25]

= √(9/25)

= 3/5

∴ sin θ = 3/5

Since, cosec θ = 1/ sin θ

= 1/ (3/5)

⇒ cosec θ = 5/3

And, sec θ = 1/ cos θ

= 1/ (4/5)

⇒ cosec θ = 5/4

Now,

tan θ = sin θ/ cos θ

= (3/5)/ (4/5)

⇒ tan θ = 3/4

And, cot θ = 1/ tan θ

= 1/ (3/4)

⇒ cot θ = 4/3

2. If sin θ = 1/√2, find all other trigonometric ratios of angle θ.

Solution:

 

We have,

sin θ = 1/√2

And we know that,

cos θ = √(1 – sin2 θ)

⇒ cos θ = √(1 – (1/√2)2)

= √(1 – (1/2))

= √[(2 – 1)/2]

= √(1/2)

= 1/√2

∴ cos θ = 1/√2

Since, cosec θ = 1/ sin θ

= 1/ (1/√2)

⇒ cosec θ = √2

And, sec θ = 1/ cos θ

= 1/ (1/√2)

⇒ sec θ = √2

Now,

tan θ = sin θ/ cos θ

= (1/√2)/ (1/√2)

⇒ tan θ = 1

And, cot θ = 1/ tan θ

= 1/ (1)

⇒ cot θ = 1

3.

Solution:

Given,

tan θ = 1/√2

By using sec2 θ − tan2 θ = 1,

4.

Solution:

Given,

tan θ = 3/4

By using sec2 θ − tan2 θ = 1,

sec θ = 5/4

Since, sec θ = 1/ cos θ

⇒ cos θ = 1/ sec θ

= 1/ (5/4)

= 4/5

So,

 

5.

Solution:

Given, tan θ = 12/5

Since, cot θ = 1/ tan θ = 1/ (12/5) = 5/12

Now, by using cosec2 θ − cot2 θ = 1

cosec θ = √(1 + cot2 θ)

= √(1 + (5/12)2 )

= √(1 + 25/144)

= √(169/ 25)

⇒ cosec θ = 13/5

Now, we know that

sin θ = 1/ cosec θ

= 1/ (13/5)

⇒ sin θ = 5/13

Putting value of sin θ in the expression we have,

= 25/ 1

= 25

6.

Solution:

Given,

cot θ = 1/√3

Using cosec2 θ − cot2 θ = 1, we can find cosec θ

cosec θ = √(1 + cot2 θ)

= √(1 + (1/√3)2)

= √(1 + (1/3)) = √((3 + 1)/3)

= √(4/3)

⇒ cosec θ = 2/√3

So, sin θ = 1/ cosec θ = 1/ (2/√3)

⇒ sin θ = √3/2

And, we know that

cos θ = √(1 – sin2 θ)

= √(1 – (√3/2)2)

= √(1 – (3/4))

= √((4 – 3)/4)

= √(1/4)

⇒ cos θ = 1/2

Now, using cos θ and sin θ in the expression, we have

= 3/5

7.

Solution:

Given,

cosec A = √2

Using cosec2 A − cot2 A = 1, we find cot A

= 4/2

= 2