RD Sharma Solutions for Class 8 Chapter 25 Data Handling - III (Pictorial Representation of Data as Pie Charts or Circle Graphs)

RD Sharma Solutions for Class 8 Chapter 25 Data Handling - III (Pictorial Representation of Data as Pie Charts or Circle Graphs) Free Online

EXERCISE 25.1 PAGE NO: 25.12

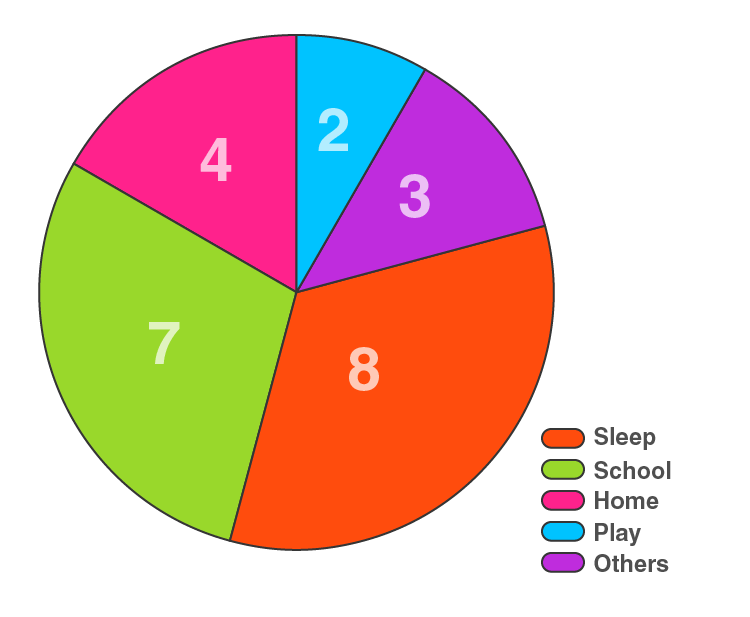
1. The number of hours, spent by a school boy on different activities in a working day, is given below:

Activities	Sleep	School	Home	Play	Others	Total
Number of Hours	8	7	4	2	3	24

Present the information in the form of a pie-chart.

Solution:

Here, total number of hours = 24

So,

The central angle = (component value/24) × 360°

The central angle for each activity will be calculated as follows

Activity	Number of Hours	Central Angle
Sleep	8	8/24 × 360° = 120o
School	7	7/24 × 360° = 105o
Home	4	4/24 × 360° = 60o
Play	2	2/24 × 360° = 30o
Others	3	3/24 × 360° = 45o

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Here, it is 120o. Construct a sector of central angle 120o whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

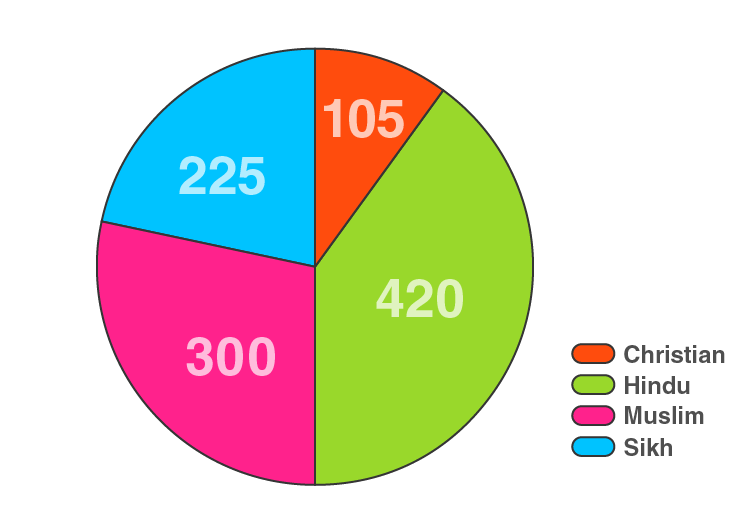
2. Employees of a company have been categorized according to their religions as given below:

Religious	Hindu	Muslim	Sikh	Christian	Total
Number of Workers	420	300	225	105	1080

Draw a pie-chart to represent the above information.

Solution:

Here, total number of workers = 1080

So,

The central angle = (component value/1080) × 360°

The central angle for each activity will be calculated as follows

Religious	Number of Workers	Central Angle
Hindu	420	420/1080 × 360° = 144
Muslim	300	300/1080 × 360° = 102.9
Sikh	225	225/1080 × 360° = 77.14
Christian	105	105/1080 × 360° = 36

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

3. In one day the sales (in rupees) of different items of a baker’s shop are given below:

Items	Ordinary bread	Fruit bread	Cakes and Pastries	Biscuits	Others	Total
Sales (in Rs)	260	40	100	60	20	780

Draw a pie-chart to represent the above information.

Solution:

Here, total sales = 780

So,

The central angle = (component value/780) × 360°

The central angle for each activity will be calculated as follows

Items	Sales (in Rs)	Central Angle
Ordinary bread	260	260/780 × 360° = 195
Fruit bread	40	40/780 × 360° = 30
Cakes and Pastries	100	100/780 × 360° = 75
Biscuits	60	60/780 × 360° = 45
Others	20	20/780 × 360° = 15

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

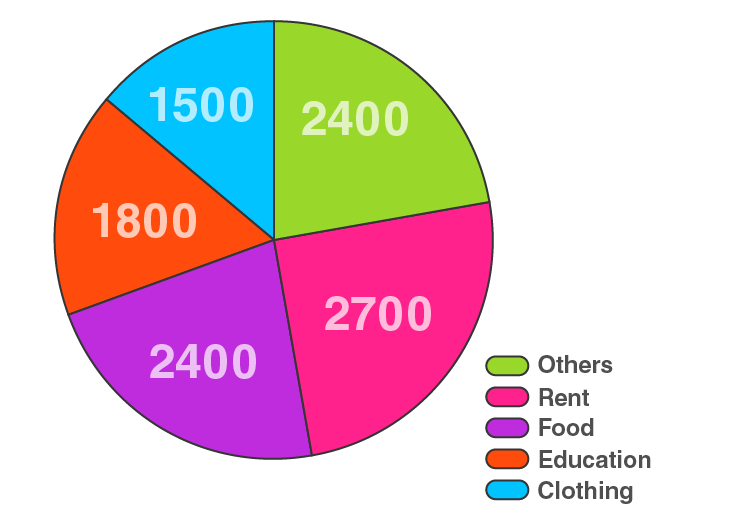
4. The following data shows the expenditure of a person on different items during a month. Represent the data by a pie-chart.

Items of expenditure	Rent	Education	Food	Clothing	Others
Amount (in Rs)	2700	1800	2400	1500	2400

Solution:

Here, total amount = Rs 10800

So,

The central angle = (component value/10800) × 360°

The central angle for each activity will be calculated as follows

Items of expenditure	Amount (in Rs)	Central angle
Rent	2700	2700/10800 × 360° = 90
Education	1800	1800/10800 × 360° = 60
Food	2400	2400/10800 × 360° = 80
Clothing	1500	1500/10800 × 360° = 50
Others	2400	2400/10800 × 360° = 80

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

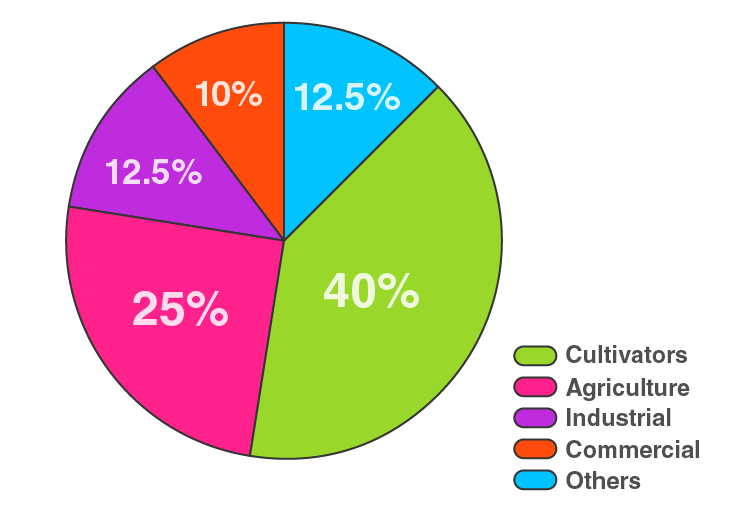
5. The percentages of various categories of workers in a state are given in the following table.

Categories	Cultivators	Agricultural Labourers	Industrial Workers	Commercial Workers	Others
% of workers	40	25	12.5	10	12.5

Present the information in the form of a pie-chart.

Solution:

Here, total % of workers = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Categories	% of workers	Central angle
Cultivators	40	400/100 × 360° = 144
Agricultural Labourers	25	25/100 × 360° = 90
Industrial Workers	12.5	12.5/100 × 360° = 45
Commercial Workers	10	10/100 × 360° = 36
Others	12.5	12.5/100 × 360° = 45

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

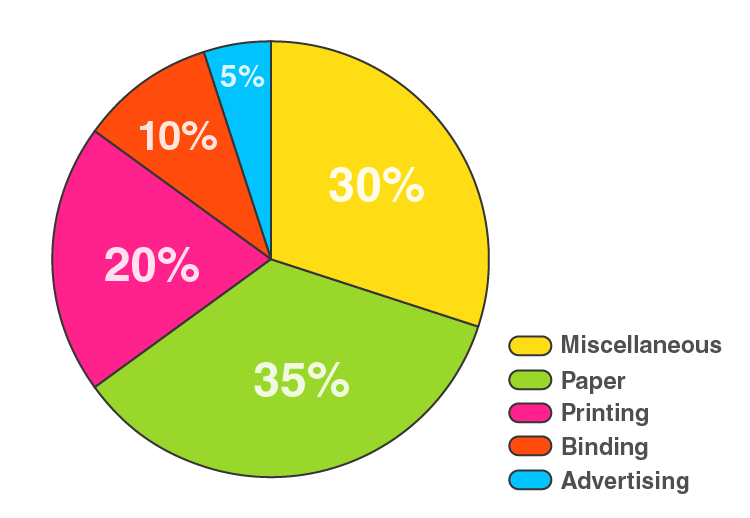
6. The following table shows the expenditure incurred by a publisher in publishing a book:

Items	Papers	Printing	Binding	Advertising	Miscellaneous
Expenditure (in %)	35%	20%	10%	5%	30%

Present the above data in the form of pie-chart.

Solution:

Here, total Expenditure (in %) = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure (in %)	Central angle
Papers	35%	35/100 × 360° = 126
Printing	20%	20/100 × 360° = 72
Binding	10%	10/100 × 360° = 36
Advertising	5%	5/100 × 360° = 18
Miscellaneous	30%	30/100 × 360° = 108

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

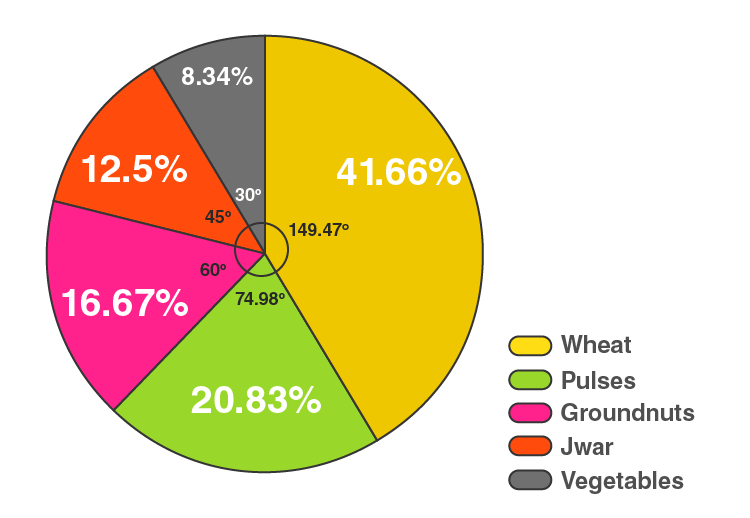
7. Percentage of the different products of a village in a particular district are given below. Draw a pie chart representing this information.

Items	Wheat	Pulses	Jwar	Groundnuts	Vegetables	Total
%	125/3	125/6	25/2	50/3	25/3	100

Solution:

Here, total % = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Items	%	Central angle
Wheat	125/3	(125/3)/100 × 360° = 150
Pulses	125/6	(125/6)/100 × 360° = 75
Jwar	25/2	(25/2)/100 × 360° = 45
Groundnuts	50/3	(50/3)/100 × 360° = 60
Vegetables	25/3	(25/3)/100 × 360° = 30

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

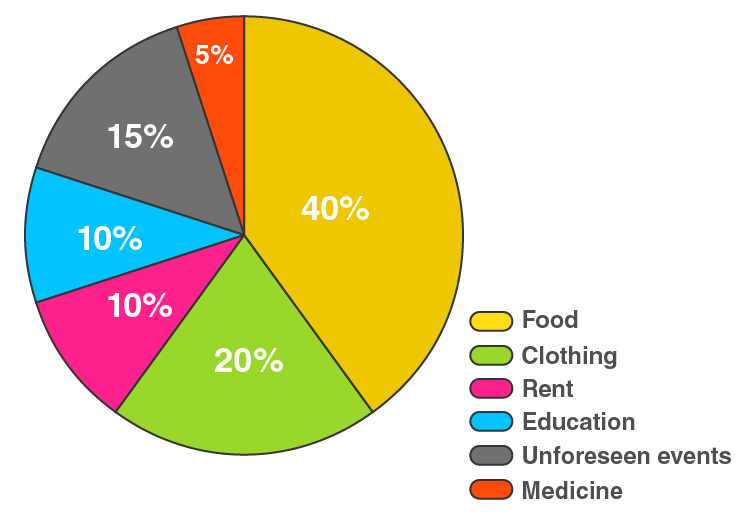
8. Draw a pie diagram for the following data of expenditure pattern in a family:

Items	Food	Clothing	Rent	Education	Unforeseen events	Medicine
Expenditure (in %)	40%	20%	10%	10%	15%	5%

Solution:

Here, total % = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure (in %)	Central angle
Food	40%	40/100 × 360° = 144
Clothing	20%	20/100 × 360° = 72
Rent	10%	10/100 × 360° = 36
Education	10%	10/100 × 360° = 36
Unforeseen events	15%	15/100 × 360° = 54
Medicines	5%	5/100 × 360° = 18

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

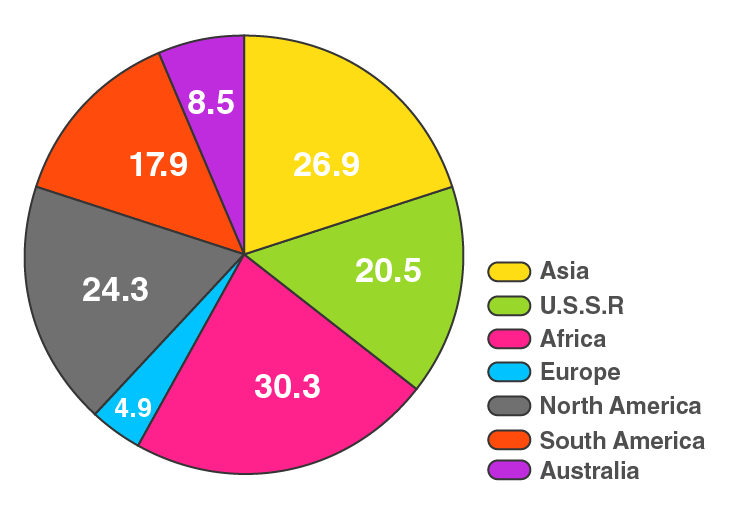
9. Draw a pie diagram of the areas of continents of the world given in the following table:

Continents	Asia	U.S.S.R	Africa	Europe	North America	South America	Australia
Area (in million sq.km)	26.9	20.5	30.3	4.9	24.3	17.9	8.5

Solution:

Here, total Area = 133.3 million sq.km

So,

The central angle = (component value/133.3) × 360°

The central angle for each activity will be calculated as follows

Continents	Area (in million sq.km)	Central angle
Asia	26.9	26.9/133.3 × 360° = 72.6
U.S.S.R	20.5	20.5/133.3 × 360° = 55.4
Africa	30.3	30.3/133.3 × 360° = 81.8
Europe	4.9	4.9/133.3 × 360° = 13.2
North America	24.3	24.3/133.3 × 360° = 65.6
South America	17.9	17.9/133.3 × 360° = 48.3
Australia	8.5	8.5/133.3 × 360° = 23

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

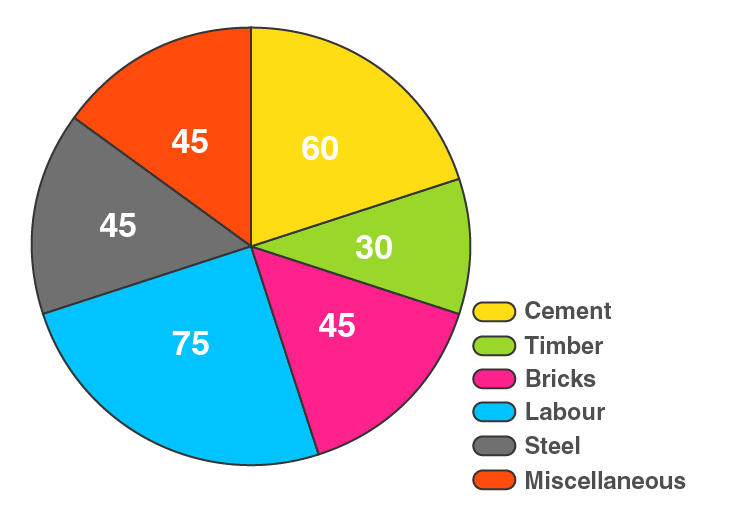
10. The following data gives the amount spent on the construction of a house. Draw a pie diagram.

Items	Cement	Timber	Bricks	Labour	steel	Miscellaneous
Expenditure (in thousand Rs)	60	30	45	75	45	45

Solution:

Here, total Expenditure = 300 thousand rupees

So,

The central angle = (component value/300) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure (in thousand Rs)	Central angle
Cement	60	60/300 × 360° = 72
Timber	30	30/300 × 360° = 36
Bricks	45	45/300 × 360° = 54
Labour	75	75/300 × 360° = 90
Steel	45	45/300 × 360° = 54
Miscellaneous	45	45/300 × 360° = 54

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

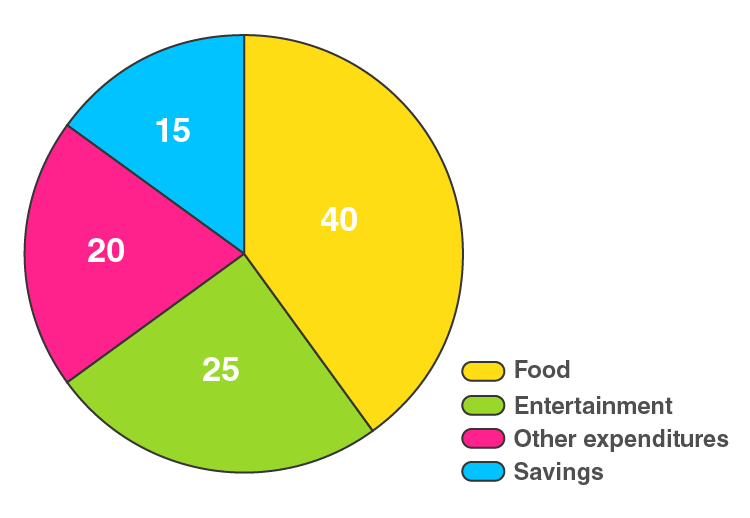
11. The following table shows how a student spends his pocket money during the course of a month. Represent it by a pie diagram.

Items	Food	Entertainment	Other Expenditure	Savings
Expenditure	40%	25%	20%	15%

Solution:

Here, total Expenditure = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure	Central angle
Food	40%	40/100 × 360° = 144
Entertainment	25%	25/100 × 360° = 90
Other Expenditure	20%	20/100 × 360° = 72
Savings	15%	15/100 × 360° = 54

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

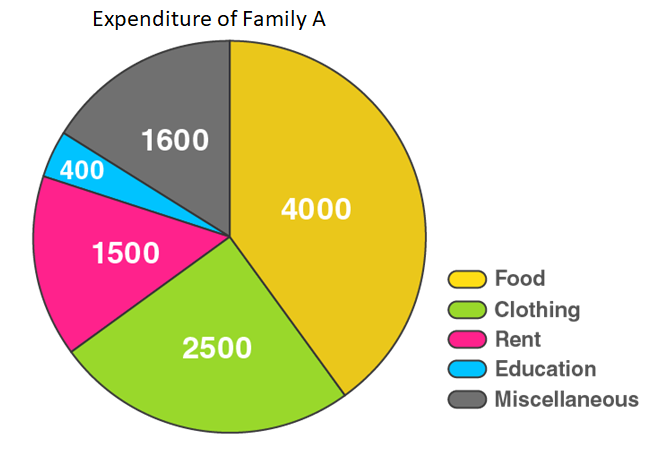
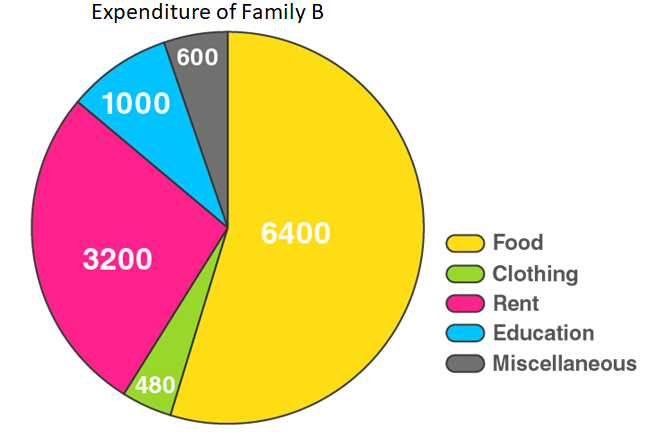
12. Represent the following data by a pie diagram:

Items of expenditure	Expenditure
	Family A Family B
Food	4000 6400
Clothing	2500 480
Rent	1500 3200
Education	400 1000
Miscellaneous	1600 600
Total	10000 16000

Solution:

Here, the total expenditure of family A = 10000 and family B = 11680

The central angle for family A = (component value/10000) × 360°

The central angle for family B = (component value/11680) × 360°

Hence, the central angle for each activity will be calculated as follows

Items of expenditure	Expenditure of Family A	Expenditure of Family B	Central angle of Family A	Central angle of Family B
Food	4000	6400	4000/10000 × 360° = 144	6400/11680 × 360° = 197.3
Clothing	2500	480	2500/10000 × 360° = 90	480/11680 × 360° = 14.8
Rent	1500	3200	1500/10000 × 360° = 54	3200/11680 × 360° = 98.6
Education	400	1000	400/10000 × 360° = 14.4	1000/11680 × 360° = 30.8
Miscellaneous	1600	600	1600/10000 × 360° = 57.6	600/11680 × 360° = 18.5

Now, the pie-chart for Family A and Family B can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

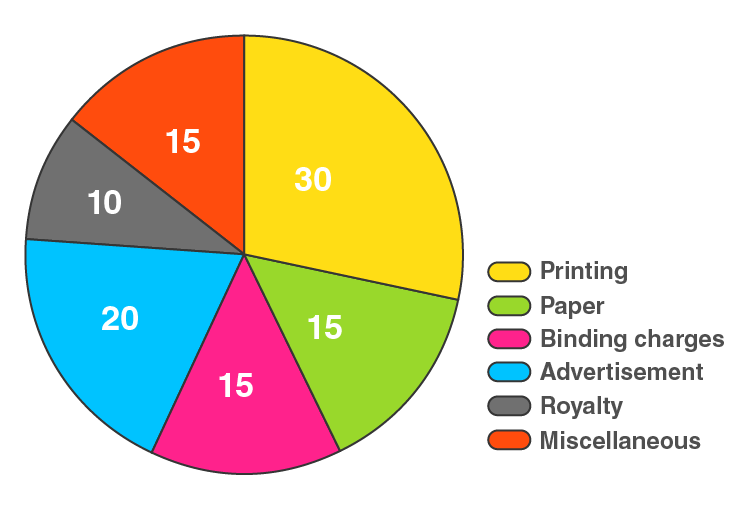
13. Following data gives the break up of the cost of production of a book:

Printing	Paper	Binding charges	Advertisement	Royalty	Miscellaneous
30%	15%	15%	20%	10%	15%

Draw a pie-diagram depicting the above information.

Solution:

Here, total cost of production of book = 105%

So,

The central angle = (component value/105) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure	Central angle
Printing	30%	30/105 × 360° = 102.9
Paper	15%	15/105 × 360° = 51.4
Binding charges	15%	15/105 × 360° = 51.4
Advertisement	20%	20/105 × 360° = 68.6
Royalty	10%	10/105 × 360° = 34.3
Miscellaneous	15%	15/105 × 360° = 51.4

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

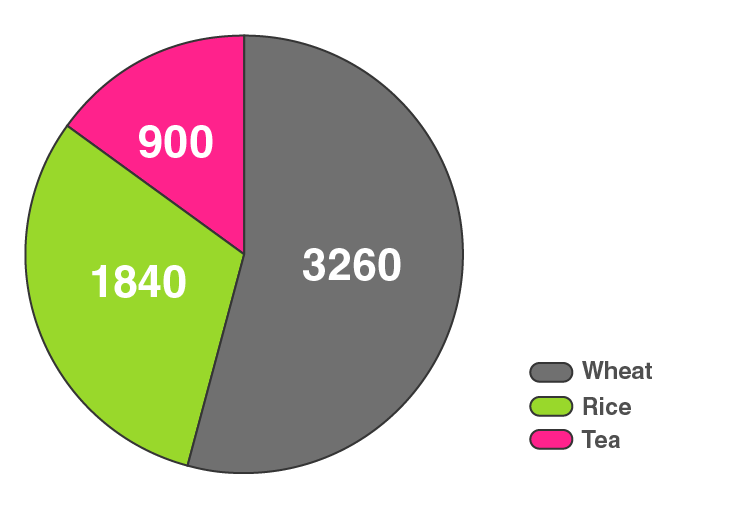
14. Represent the following data with the help of pie diagram:

Items	Wheat	Rice	Tea
Production (in metric tons)	3260	1840	900

Solution:

Here, total cost of production = 6000 metric tons

So,

The central angle = (component value/6000) × 360°

The central angle for each activity will be calculated as follows

Items	Production	Central angle
Wheat	3260	3260/6000 × 360° = 195.6
Rice	1840	1840/6000 × 360° = 110.4
Tea	900	900/6000 × 360° = 54

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

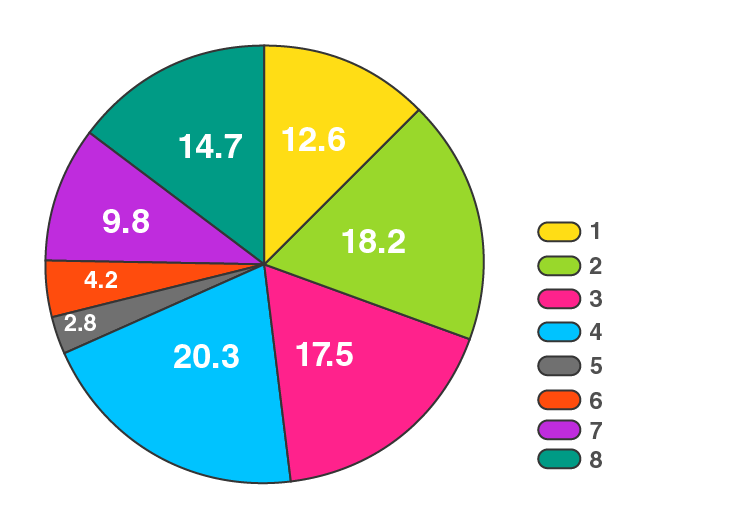
15. Draw a pie-diagram representing the relative frequencies (expressed as percentage) of the eight classes as given below:
12.6, 18.2, 17.5, 20.3, 2.8, 4.2, 9.8, 14.7

Solution:

Here, total amount = 100.1%

So,

The central angle = (component value/100.1) × 360°

The central angle for each activity will be calculated as follows

Class	Amount (in %)	Central angle
1	12.6	12.6/100.1 × 360° = 45.3
2	18.2	18.2/100.1 × 360° = 65.5
3	17.5	17.5/100.1 × 360° = 62.9
4	20.3	20.3/100.1 × 360° = 73
5	2.8	2.8/100.1 × 360° = 10.1
6	4.2	4.2/100.1 × 360° = 15.1
7	9.8	9.8/100.1 × 360° = 35.2
8	14.7	14.7/100.1 × 360° = 52.9

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

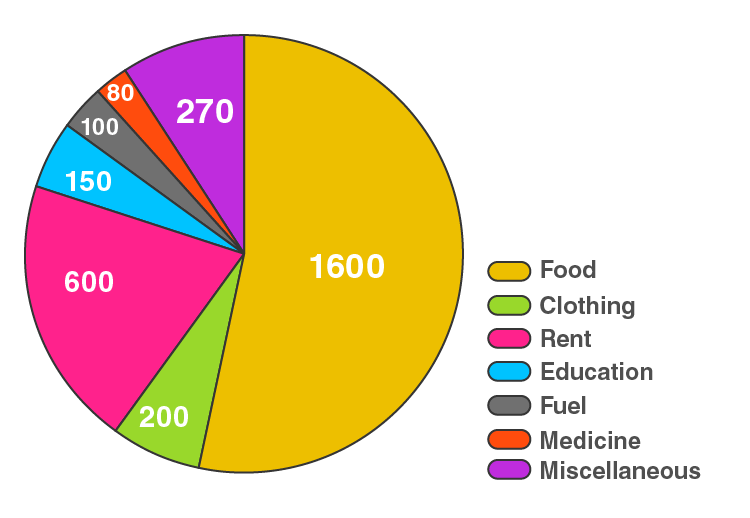
16. Following is the break up of the expenditure of a family on different items of consumption:

Items	Food	Clothing	Rent	Education	Fuel etc.	Medicine	Miscellaneous
Expenditure (in Rs)	1600	200	600	150	100	80	270

Draw a pie-diagram to represent the above data.

Solution:

Here, total expenditure = 3000 Rs

So,

The central angle = (component value/3000) × 360°

The central angle for each activity will be calculated as follows

Items	Expenditure (in Rs)	Central angle
Food	1600	1600/3000 × 360° = 192
Clothing	200	200/3000 × 360° = 24
Rent	600	600/3000 × 360° = 72
Education	150	150/3000 × 360° = 18
Fuel	100	100/3000 × 360° = 12
Medicine	80	80/3000 × 360° = 9.6
Miscellaneous	270	270/3000 × 360° = 32.4

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

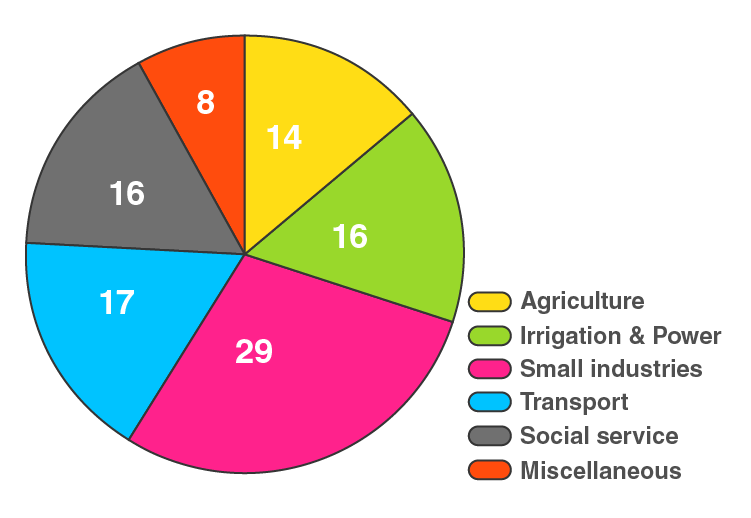
17. Draw a pie diagram for the following data of the investment pattern in a five years plan:

Agriculture	Irrigation and Power	Small Industries	Transport	Social service	Miscellaneous
14%	16%	29%	17%	16%	8%

Solution:

Here, total investment = 100%

So,

The central angle = (component value/100) × 360°

The central angle for each activity will be calculated as follows

Data	Investment	Central angle
Agriculture	14%	14/100 × 360° = 50.4
Irrigation and Power	16%	16/100 × 360° = 57.6
Small Industries	29%	29/100 × 360° = 104.4
Transport	17%	17/100 × 360° = 61.2
Social service	16%	16/100 × 360° = 57.6
Miscellaneous	8%	8/100 × 360° = 28.8

Now, the pie-chart can be constructed by using the given data.

Steps to construct:

Step 1: Draw the circle of an appropriate radius.

Step 2: Draw a vertical radius anywhere inside the circle.

Step 3: Choose the largest central angle. Construct a sector of central angle, whose one radius coincides with the radius drawn in step 2 and the other radius is in clockwise direction to the vertical radius.

Step 4: Construct other sectors representing other values in clockwise direction in descending order of magnitudes of their central angles.

Step 5: Shade the sectors so obtained by different colours and label them as shown in below figure.

EXERCISE 25.2 PAGE NO: 25.21

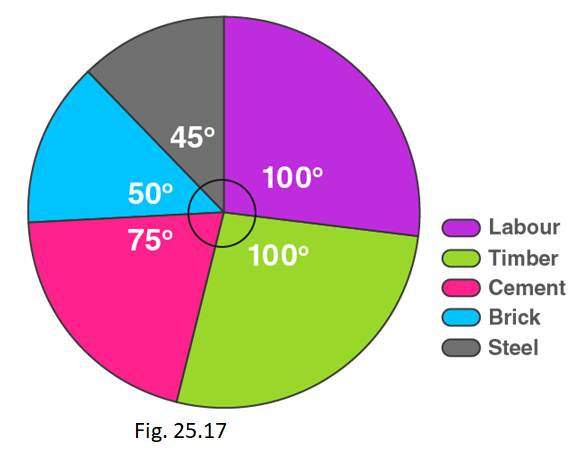
1. The pie chart given in Fig. 25.17 represents the expenditure on different items in constructing a flat in Delhi. If the expenditure incurred on cement is Rs. 112500, find the following:

(i) Total cost of the flat.
(ii) Expenditure incurred on labour.

Solution:

(i) By using the formula,

Expenditure incurred on cement = (central angle × Total cost) / 360°

Total cost of the flat = (360° × 112500) / 75o = Rs 540000

(ii) By using the formula,

Expenditure incurred on labour = (central angle × Total cost) / 360°

= (100° × 540000) / 360o = Rs 150000

2. The pie-chart given in Fig. 25.18 shows the annual agricultural production of an Indian state. If the total production of all the commodities is 81000 tonnes, find the production (in tonnes) of

(i) Wheat (ii) Sugar (iii) Rice (iv) Maize (v) Gram

Solution:

We know that,

Total Production = 81000 Tonnes.

So,

(i) Production of wheat = (central angle of wheat × Total production) / 360°

= (120o × 81000) / 360o = 27000 tonnes

(ii) Production of sugar = (central angle of sugar × Total production) / 360°

= (100o × 81000) / 360o = 22500 tonnes

(iii) Production of rice = (central angle of rice × Total production) / 360°

= (60o × 81000) / 360o = 13500 tonnes

(iv) Production of maize = (central angle of maize × Total production) / 360°

= (30o × 81000) / 360o = 6750 tonnes

(v) Production of gram = (central angle of gram × Total production) / 360°

= (50o × 81000) / 360o = 11250 tonnes

3. The following pie chart shows the number of students admitted in different faculties of a college. If 1000 students are admitted in Science answer the following :

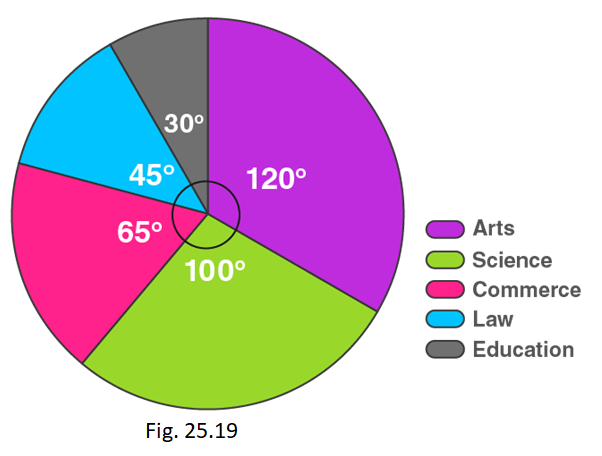
(i) What is the total number of students?
(ii) What is the ratio of students in science and arts?

Solution:

(i) 

Students in science = (central angle × Total students) / 360°

1000 = (100o × Total students) / 360o

Total students = (1000 × 360o)/100o

= 3600 students

∴ Total number of students are 3600.

(ii) Students in arts = (central angle of arts × Total students) / 360°

= (120o × 3600) / 360o = 1200 students

∴ Ratio of students in science and arts is 1000:1200 = 5:6

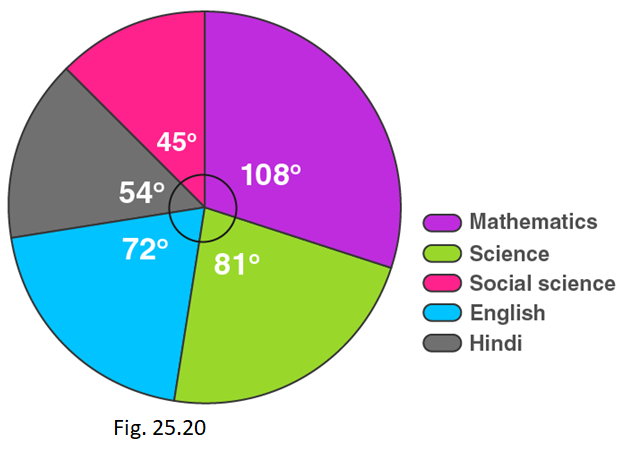
4. In Fig. 25.20, the pie-chart shows the marks obtained by a student in an examination. If the student secures 440 marks in all, calculate his marks in each of the given subjects.

Solution:

Marks secured in mathematics = (central angle of maths × Total score secured) / 360°

= (108 × 440) / 360o = 132 marks

Marks secured in science = (central angle of science × Total score secured) / 360°

= (81 × 440) / 360o = 99 marks

Marks secured in English = (central angle of English × Total score secured) / 360°

= (72 × 440) / 360o = 88 marks

Marks secured in Hindi = (central angle of Hindi × Total score secured) / 360°

= (54 × 440) / 360o = 66 marks

Marks secured in social science = (central angle of social science × Total score secured) / 360°

= (45 × 440) / 360o = 55 marks

Subject	Mathematics	Science	English	Hindi	Social Science
Marks secured	132	99	88	66	55

5. In Fig. 25.21, the pie chart shows the marks obtained by a student in various subjects. If the student scored 135 marks in mathematics, find the total marks in all the subjects. Also, find his score in individual subjects.

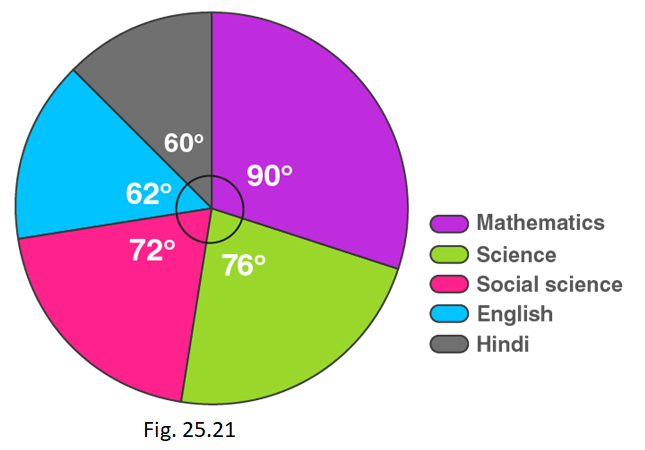
Solution:

Let us calculate the total marks.

So,

Marks scored in mathematics = (central angle of maths × Total marks) / 360°

135 = (90 × Total marks) / 360o

Total marks = (135 × 360)/90

= 540 marks

Now,

Marks scored in Hindi = (central angle of Hindi × Total marks) / 360°

= (60 × 540) / 360o

= 90 marks

Marks scored in Science = (central angle of Science × Total marks) / 360°

= (76 × 540) / 360o

= 114 marks

Marks scored in Social science = (central angle of Social science × Total marks) / 360°

= (72 × 540) / 360o

= 108 marks

Marks scored in English = (central angle of English × Total marks) / 360°

= (62 × 540) / 360o

= 93 marks

Subject	Mathematics	Science	Social science	English	Hindi
Marks secured	135	114	108	93	90

6. The following pie chart shows the monthly expenditure of Shikha on various items. If she spends Rs 16000 per month, answer the following questions:

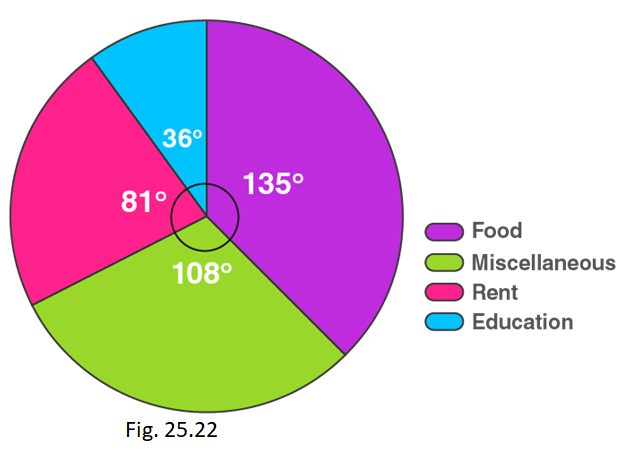
(i) How much does she spend on rent?

(ii) How much does she spend on education?

(iii) What is the ratio of expenses on food and rent?

Solution:

(i) Money spent on rent = (central angle of rent × Total money spent) / 360°

= (81 × 16000) / 360o

= Rs 3600

(ii) Money spent on education = (central angle of education × Total money spent) / 360°

= (36 × 16000) / 360o

= Rs 1600

(iii) Money spent on food = (central angle of food × Total money spent) / 360°

= (135 × 16000) / 360o

= Rs 6000

Ratio of expenses on food and rent is Rs 6000/Rs3600 = 5/3

Ratio = 5:3

7. The pie chart (as shown in the figure 25.23) represents the amount spent on different sports by a sports club in a year. If the total money spent by the club on sports is Rs 108000, find the amount spent on each sport.

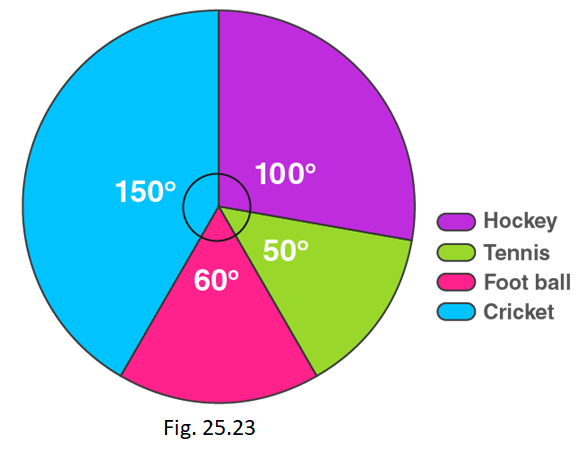
Solution:

Money spent on cricket = (central angle of cricket × Total money spent) / 360°

= (150 × 108000) / 360o

= Rs 45000

Money spent on football = (central angle of football × Total money spent) / 360°

= (60 × 108000) / 360o

= Rs 18000

Money spent on tennis = (central angle of tennis × Total money spent) / 360°

= (50 × 108000) / 360o

= Rs 15000

Money spent on hockey = (central angle of cricket × Total money spent) / 360°

= (100 × 108000) / 360o

= Rs 30000