RD Sharma Solutions for Class 8 Chapter 20 Mensuration - I (Area of a Trapezium and a Polygon)

RD Sharma Solutions for Class 8 Chapter 20 Mensuration - I (Area of a Trapezium and a Polygon) Free Online

EXERCISE 20.1 PAGE NO: 20.13

1. A flooring tile has the shape of a parallelogram whose base is 24 cm and the corresponding height is 10 cm. How many such tiles are required to cover a floor of area 1080 m2?

Solution:

Given that,

Base of parallelogram = 24cm

Height of parallelogram = 10cm

Area of floor = 1080m2

We know that,

Area of parallelogram = Base × Height

Area of 1 tile = 24 × 10 = 240cm2

We know that, 1m = 100cm

So for 1080m2 = 1080 × 100 × 100 cm2

To calculate the Number of tiles required = Area of floor/Area of 1 tile

i.e., Number of tiles required = (1080 × 100 × 100) / (24 × 10) = 45000

∴ Number of tiles required = 45000

2. A plot is in the form of a rectangle ABCD having semi-circle on BC as shown in Fig. 20.23. If AB = 60 m and BC = 28 m, Find the area of the plot.

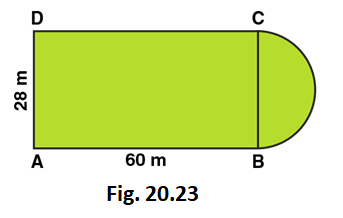
Solution:

Area of the plot = Area of the rectangle + Area of semi-circle

Radius of semi-circle = BC/2 = 28/2 = 14m

Area of the Rectangular plot = Length × Breadth = 60 × 28 = 1680 m2

Area of the Semi-circular portion = πr2/2

= 1/2 × 22/7 × 14 × 14

= 308 m2

∴ The total area of the plot = 1680 + 308 = 1988 m2

3. A playground has the shape of a rectangle, with two semi-circles on its smaller sides as diameters, added to its outside. If the sides of the rectangle are 36 m and 24.5 m, find the area of the playground. (Take π= 22/7.)

Solution:

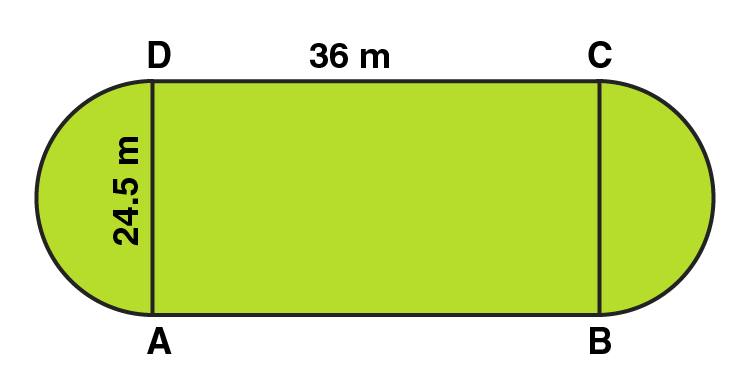
Area of the plot = Area of the Rectangle + 2 × area of one semi-circle

Radius of semi-circle = BC/2 = 24.5/2 = 12.25m

Area of the Rectangular plot = Length × Breadth = 36 × 24.5 = 882 m2

Area of the Semi-circular portions = 2 × πr2/2

= 2 × 1/2 × 22/7 × 12.25 × 12.25 = 471.625 m2

Area of the plot = 882 + 471.625 = 1353.625 m2

4. A rectangular piece is 20 m long and 15 m wide. From its four corners, quadrants of radii 3.5 m have been cut. Find the area of the remaining part.

Solution:

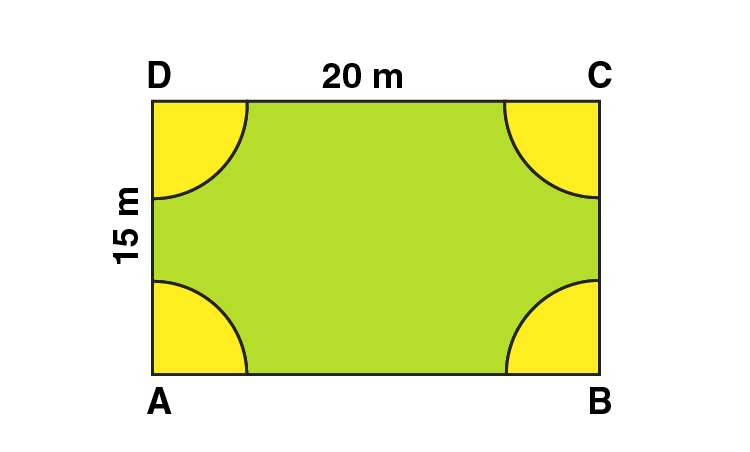
Area of the plot = Area of the rectangle – 4 × area of one quadrant

Radius of semi-circle = 3.5 m

Area of four quadrants = area of one circle

Area of the plot = Length × Breadth – πr2

Area of the plot = 20 × 15 – (22/7 × 3.5 × 3.5)

Area of the plot = 300 – 38.5 = 261.5 m2

5. The inside perimeter of a running track (shown in Fig. 20.24) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If track is everywhere 14 m wide, find the area of the track. Also, find the length of the outer running track.

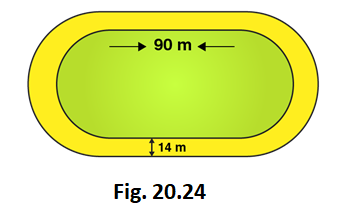
Solution:

Perimeter of the inner track = 2 × Length of rectangle + perimeter of two semi-circular ends

Perimeter of the inner track = Length + Length + 2πr

400 = 90 + 90 + (2 × 22/7 × r)

(2 × 22/7 × r) = 400 – 180

(2 × 22/7 × r) = 220

44r = 220 × 7

44r = 1540

r = 1540/44 = 35

r = 35m

So, the radius of inner circle = 35 m

Now, let’s calculate the radius of outer track

Radius of outer track = Radius of inner track + width of the track

Radius of outer track = 35 + 14 = 49m

Length of outer track = 2× Length of rectangle + perimeter of two outer semi-circular ends

Length of outer track = 2× 90 + 2πr

Length of outer track = 2× 90 + (2 × 22/7 × 49)

Length of outer track = 180 + 308 = 488

So, Length of outer track = 488m

Area of inner track = Area of inner rectangle + Area of two inner semi-circles

Area of inner track = Length × Breadth + πr2

Area of inner track = 90 × 70 + (22/7 × 35 × 35)

Area of inner track = 6300 + 3850

So, Area of inner track = 10150 m2

Area of outer track = Area of outer rectangle + Area of two outer semi-circles

Breadth of outer track = 35 + 35 +14 + 14 = 98 m

Area of outer track = length× breadth + πr2

Area of outer track = 90 × 98 + (22/7 × 49 × 49)

Area of outer track = 8820 + 7546

So, Area of outer track = 16366 m2

Now, let’s calculate area of path

Area of path = Area of outer track – Area of inner track

Area of path = 16366 – 10150 = 6216

So, Area of path = 6216 m2

6. Find the area of Fig. 20.25, in square cm, correct to one place of decimal. (Take π =22/7)

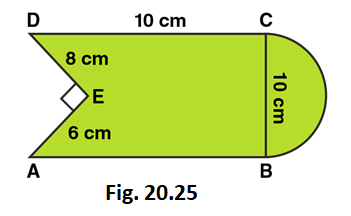
Solution:

Area of the Figure = Area of square + Area of semi-circle – Area of right angled triangle

Area of the Figure = side × side + πr2/2 – (1/2 × base × height)

Area of the Figure = 10 × 10 + (1/2 × 22/7 × 5 × 5) – (1/2 × 8 × 6)

Area of the Figure = 100 + 39.28 – 24

Area of the Figure = 115.3

So, Area of the Figure = 115.3 cm2

7. The diameter of a wheel of a bus is 90 cm which makes 315 revolutions per minute. Determine its speed in kilometres per hour. (Take π=22/7)

Solution:

Given that, Diameter of a wheel = 90 cm

We know that, Perimeter of wheel = 2πd

Perimeter of wheel = 22/7 × 90 = 282.857

So, Perimeter of a wheel = 282.857 cm

Distance covered in 315 revolutions = 282.857× 315 = 89099.955 cm

One km = 100000 cm

Therefore, Distance covered = 89099.955/100000 = 0.89 km

Speed in km per hour = 0.89 × 60 = 53.4 km per hour

8. The area of a rhombus is 240 cm2 and one of the diagonal is 16 cm. Find another diagonal.

Solution:

Area of rhombus = 1/2 × d1 × d2

240 = 1/2 × 16 × d2

240 = 8 × d2

d2 = 240/8 = 30

So, the other diagonal is 30 cm

9. The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.

Solution:

Area of rhombus = 1/2 × d1 × d2

Area of rhombus = 1/2 × 7.5 × 12

Area of rhombus = 6 × 7.5 = 45

So, Area of rhombus = 45 cm2

10. The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.

Solution:

Area of quadrilateral = 1/2 × d1 × (p1 + p2)

Area of quadrilateral = 1/2 × 24 × (8 + 13)

Area of quadrilateral = 12 × 21 = 252

So, Area of quadrilateral is 252 cm2

11. Find the area of a rhombus whose side is 6 cm and whose altitude is 4 cm. If one of its diagonals is 8 cm long, find the length of the other diagonal.

Solution:

Given that,

Side of rhombus = 6 cm

Altitude of rhombus = 4 cm

Since rhombus is a parallelogram, therefore area of parallelogram = base × altitude

i.e., Area of parallelogram = 6 × 4 = 24 cm2

Area of parallelogram = Area of rhombus

Area of rhombus = 1/2 × d1 × d2

24 = 1/2 × 8 × d2

24 = 4 × d2

d2 = 24/4 = 6

So, length of other diagonal of rhombus is 6 cm

12. The floor of a building consists of 3000 tiles which are rhombus shaped and each of its diagonals are 45 cm and 30 cm in length. Find the total cost of polishing the floor, if the cost per m2 is Rs. 4.

Solution:

We know that,

Area of rhombus = 1/2 × d1 × d2

Area of rhombus = 1/2 × 45 × 30

Area of rhombus = 1350/2 = 675

So, Area of rhombus = 675 cm2

∴ Area of one tile = 675 cm2

Now, Area of 3000 tiles = 675× 3000 = 2025000 cm2

Area of tiles in m2 = 2025000/10000 = 202.5 m2

Total cost for polishing the floor = 202.5× 4 = Rs 810

13. A rectangular grassy plot is 112 m long and 78 m broad. It has gravel path 2.5 m wide all around it on the side. Find the area of the path and the cost of constructing it at Rs. 4.50 per square metre.

Solution:

We know that,

Inner area of rectangle = length × breadth

Inner area of rectangle = 112 × 78 = 8736 m2

Width of path = 2.5 m

Length of outer rectangle = 112 – (2.5 + 2.5) = 107 m

Breadth of outer rectangle = 78 – (2.5 + 2.5) = 73 m

And,

Outer area of rectangle = length × breadth

Outer area of rectangle = 107 × 73 = 7811 m2

Now let’s calculate Area of path,

Area of path = Outer area of rectangle – Inner area of rectangle

Area of path = 8736 – 7811 = 925 m2

Also given that,

Cost of construction for 1 m2 = Rs 4.50

∴ Cost of construction for 925 m2 = 925 × 4.50 = Rs 4162.5

14. Find the area of a rhombus, each side of which measures 20 cm and one of whose diagonals is 24 cm.

Solution:

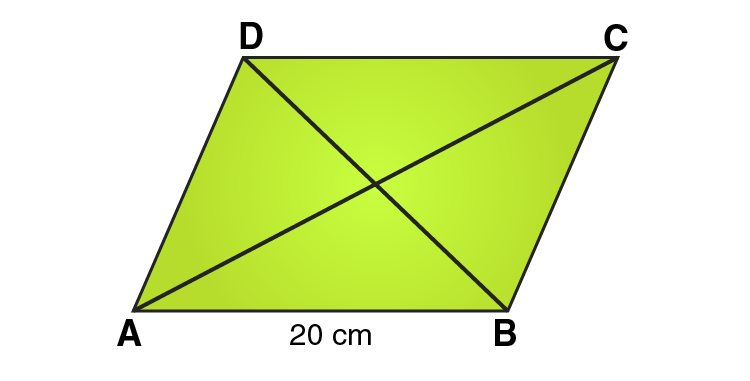
Given that,

Length of side of rhombus = 20 cm

Length of one diagonal = 24 cm

In ΔAOB,

Using Pythagoras theorem:

AB2 = OA2 + OB2

202 = 122 + OB2

OB2 = 202 – 122

OB2 = 400 – 144

OB2 = 256

OB = 16

So, length of the other diameter = 16 × 2 = 32 cm

Area of rhombus = 1/2 × d1 × d2

Area of rhombus = 1/2 × 24 × 32

Area of rhombus = 384 cm2

15. The length of a side of a square field is 4 m. What will be the altitude of the rhombus, if the area of the rhombus is equal to the square field and one of its diagonal is 2 m?

Solution:

Given that,

Length of a side of a square = 4 m

Area of square = side2

Area of square = 4 × 4 = 16 m2

We know that,

Area of square = Area of rhombus

So, Area of rhombus = 16 m2

Area of rhombus = 1/2 × d1 × d2

16 = 1/2 × 2 × d2

16 = d2

∴ the diagonal of rhombus = 16 m

In ΔAOB,

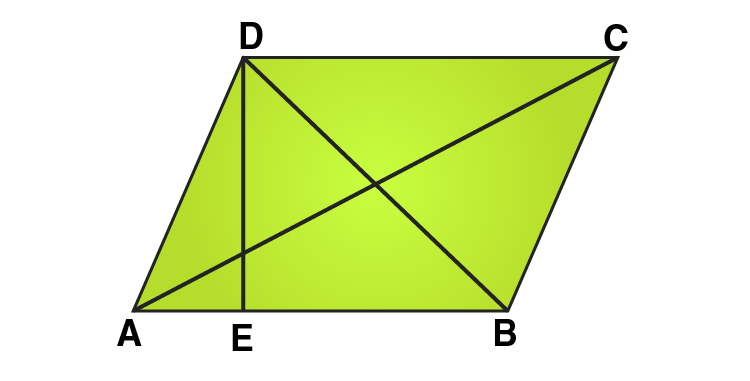
Using Pythagoras theorem:

AB2 = OA2 + OB2

AB2 = 82 + 12

AB2 = 65

AB = √65

Since rhombus is a parallelogram, therefore area of parallelogram = base × altitude

Area of parallelogram = AB × DE

16 = √65 × DE

DE = 16/√65

i.e., Altitude of Rhombus = 16/√65 cm

16. Find the area of the field in the form of a rhombus, if the length of each side be 14 cm and the altitude be 16 cm.

Solution:

Given that,

Side of rhombus = 14 cm

Altitude of rhombus = 16 cm

Since rhombus is a parallelogram, therefore

Area of parallelogram = base × altitude

Area of parallelogram = 14 × 16 = 224 cm2

17. The cost of fencing a square field at 60 paise per metre is Rs. 1200. Find the cost of reaping the field at the rate of 50 paise per 100 sq. metres.

Solution:

Perimeter of square field = Cost of fencing / rate of fencing

Perimeter of square field = 1200/0.6 = 2000

So, Perimeter of square field = 2000 m

Perimeter of square = 4 × side

Side of square = Perimeter / 4 = 2000/4 = 500

So, Side of square = 500 m

We know that, Area of square = side2

Area of square = 500 × 500 = 250000 m2

Cost of reaping = (250000 × 0.5) / 100 = 1250

∴ Cost of reaping the field is Rs 1250

18. In exchange of a square plot one of whose sides is 84 m, a man wants to buy a rectangular plot 144 m long and of the same area as of the square plot. Find the width of the rectangular plot.

Solution:

Area of square = side2

Area of square = 84 × 84 = 7056

Since, Area of square = Area of rectangle

7056 = 144 × width

Width = 7056/144 = 49

∴ Width of rectangle = 49 m

19. The area of a rhombus is 84 m2. If its perimeter is 40 m, then find its altitude.

Solution:

Given that,

Area of rhombus = 84 m2

Perimeter = 40 m

We know that,

Perimeter of rhombus = 4 × side

∴ Side of rhombus = Perimeter / 4 = 40/4 = 10

So, Side of rhombus = 10 m

Since rhombus is a parallelogram, therefore Area of parallelogram = base × altitude

84 = 10 × altitude

Altitude = 84/10 = 8.4

So, Altitude of rhombus = 8.4 m

20. A garden is in the form of a rhombus whose side is 30 metres and the corresponding altitude is 16 m. Find the cost of levelling the garden at the rate of Rs. 2 per m2.

Solution:

Given that,

Side of rhombus = 30 m

Altitude of rhombus = 16 m

Since rhombus is a parallelogram, therefore Area of parallelogram = base × altitude

Area of parallelogram = 30 × 16 = 480 m2

Cost of levelling the garden = area × rate

Cost of levelling the garden = 480 × 2 = 960

So, Cost of levelling the garden is Rs 960

21. A field in the form of a rhombus has each side of length 64 m and altitude 16 m. What is the side of a square field which has the same area as that of a rhombus?

Solution:

Given that,

Side of rhombus = 64 m

Altitude of rhombus = 16 m

Since rhombus is a parallelogram, therefore Area of parallelogram = base × altitude

Area of parallelogram = 64 × 16 = 1024 m2

Since Area of rhombus = Area of square

Therefore, Area of square = side2

Or side2 = Area of square

Side of a square = √square

Side of square = √1024 = 32

∴ Side of square = 32 m

22. The area of a rhombus is equal to the area of a triangle whose base and the corresponding altitude are 24.8 cm and 16.5 cm respectively. If one of the diagonals of the rhombus is 22 cm, find the length of the other diagonal.

Solution:

Given that,

Length of base of triangle = 24.8 cm

Length of altitude of triangle= 16.5 cm

∴ Area of triangle = 1/2 × base × altitude

Area of triangle = 1/2 × 24.8 × 16.5 = 204.6

So, Area of triangle = 204.6 cm

Since, Area of triangle = Area of rhombus

∴ Area of rhombus = 1/2 × d1 × d2

204.6 = 1/2 × 22 × d2

204.6 = 11 × d2

d2 = 204.6/11 = 18.6

∴ The length of other diagonal is 18.6 cm

---

EXERCISE 20.2 PAGE NO: 20.22

1. Find the area, in square metres, of the trapezium whose bases and altitudes are as under:
(i) bases = 12 dm and 20 dm, altitude = 10 dm
(ii) bases = 28 cm and 3 dm, altitude = 25 cm
(iii) bases = 8 m and 60 dm, altitude = 40 dm
(iv) bases = 150 cm and 30 dm, altitude = 9 dm

Solution:

(i) Given that,

Length of bases of trapezium = 12 dm and 20 dm

Length of altitude = 10 dm

We know that, 10 dm = 1 m

∴ Length of bases in m = 1.2 m and 2 m

Similarly, length of altitude in m = 1 m

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (1.2 + 2.0) × 1

Area of trapezium = 1/2 × 3.2 = 1.6

So, Area of trapezium = 1.6m2

(ii) Given that,

Length of bases of trapezium = 28 cm and 3 dm

Length of altitude = 25 cm

We know that, 10 dm = 1 m

∴ Length of bases in m = 0.28 m and 0.3 m

Similarly, length of altitude in m = 0.25 m

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (0.28 + 0.3) × 0.25

Area of trapezium = 1/2 × 0.58× 0.25 = 0.0725

So, Area of trapezium = 0.0725m2

(iii) Given that,

Length of bases of trapezium = 8 m and 60 dm

Length of altitude = 40 dm

We know that, 10 dm = 1 m

∴ Length of bases in m = 8 m and 6 m

Similarly, length of altitude in m = 4 m

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (8 + 6) × 4

Area of trapezium = 1/2 × 56 = 28

So, Area of trapezium = 28m2

(iv) Given that,

Length of bases of trapezium = 150 cm and 30 dm

Length of altitude = 9 dm

We know that, 10 dm = 1 m

∴ Length of bases in m = 1.5 m and 3 m

Similarly, length of altitude in m = 0.9 m

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (1.5 + 3) × 0.9

Area of trapezium = 1/2 × 4.5 × 0.9 = 2.025

So, Area of trapezium = 2.025m2

2. Find the area of trapezium with base 15 cm and height 8 cm, if the side parallel to the given base is 9 cm long.

Solution:

Given that,

Length of bases of trapezium = 15 cm and 9 cm

Length of altitude = 8 cm

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (15 + 9) × 8

Area of trapezium = 1/2 × 192 = 96

So, Area of trapezium = 96m2

3. Find the area of a trapezium whose parallel sides are of length 16 dm and 22 dm and whose height is 12 dm.

Solution:

Given that,

Length of bases of trapezium = 16 dm and 22 dm

Length of altitude = 12 dm

We know that, 10 dm = 1 m

∴ Length of bases in m = 1.6 m and 2.2 m

Similarly, length of altitude in m = 1.2 m

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of trapezium = 1/2 (1.6 + 2.2) × 1.2

Area of trapezium = 1/2 × 3.8 × 1.2 = 2.28

So, Area of trapezium = 2.28m2

4. Find the height of a trapezium, the sum of the lengths of whose bases (parallel sides) is 60 cm and whose area is 600 cm2.

Solution:

Given that,

Length of bases of trapezium = 60 cm

Area = 600 cm2

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

600 = 1/2 (60) × altitude

600 = 30 × altitude

Which implies, altitude = 600/30 = 20

∴ Length of altitude is 20 cm

5. Find the altitude of a trapezium whose area is 65 cm2 and whose base are 13 cm and 26 cm.

Solution:

Given that,

Length of bases of trapezium = 13 cm and 26 cm

Area = 65 cm2

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

65 = 1/2 (13 + 26) × altitude

65 = 39/2 × altitude

Which implies, altitude = (65×2) /39 = 130/39 = 10/3

∴ Length of altitude = 10/3 cm

6. Find the sum of the lengths of the bases of a trapezium whose area is 4.2 m2 and whose height is 280 cm.

Solution:

Given that,

Height of trapezium = 280 cm = 2.8m

Area = 4.2 m2

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude                                    To calculate the length of parallel sides we can rewrite the above equation as,

Sum of lengths of parallel sides = (2 × Area) / altitude

Sum of lengths of parallel sides = (2 × 4.2) / 2.8 = 8.4/2.8 = 3

∴ Sum of lengths of parallel sides = 3 m

7. Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as,
(i) the sum of the areas of two triangles and one rectangle.
(ii) the difference of the area of a rectangle and the sum of the areas of two triangles.

Solution:

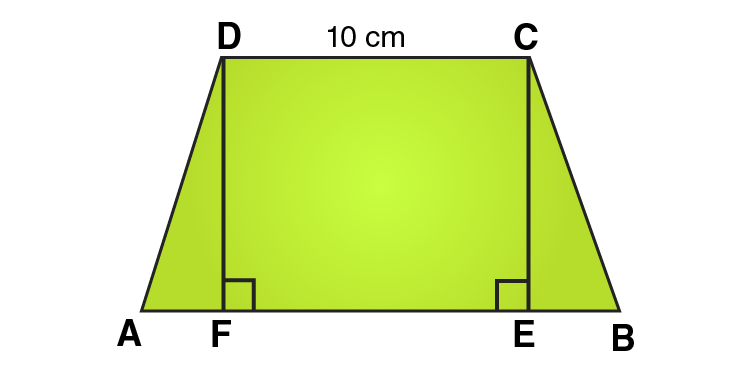
We know that, Area of a trapezium ABCD

= area (∆DFA) + area (rectangle DFEC) + area (∆CEB)

= (1/2 × AF × DF) + (FE × DF) + (1/2 × EB × CE)

= (1/2 × AF × h) + (FE × h) + (1/2 × EB × h)

= 1/2 × h × (AF + 2FE + EB)

= 1/2 × h × (AF + FE + EB + FE)

= 1/2 × h × (AB + FE)

= 1/2 × h × (AB + CD) [Opposite sides of rectangle are equal]

= 1/2 × 6 × (15 + 10)

= 1/2 × 6 × 25 = 75

∴ Area of trapezium = 75 cm2

8. The area of a trapezium is 960 cm2. If the parallel sides are 34 cm and 46 cm, find the distance between them.

Solution:

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

To calculate the distance between parallel sides we can rewrite the above equation as,

Distance between parallel sides = (2 × Area) / Sum of sides

= (2 × 960) / (34 + 46)

= (2 × 960) / 80 = 1920/80 = 24

∴ Distance between parallel sides = 24 cm

9. Find the area of Fig. 20.35 as the sum of the areas of two trapezium and a rectangle.

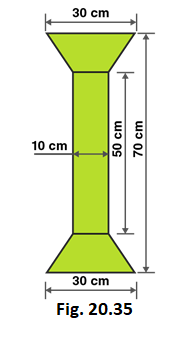
Solution:

From the figure we can write,

Area of figure = Area of two trapeziums + Area of rectangle

Given that,

Length of rectangle = 50 cm

Breadth of rectangle = 10 cm

Length of parallel sides of trapezium = 30 cm and 10 cm

Distance between parallel sides of trapezium = (70–50)/2 = 20/2 = 10

So, Distance between parallel sides of trapezium = 10 cm

Area of figure = 2 × 1/2 (Sum of lengths of parallel sides) × altitude + Length × Breadth

Area of figure = 2 × 1/2 (30+10) × 10 + 50 × 10

Area of figure = 40 × 10 + 50 × 10

Area of figure = 400 + 500 = 900

∴ Area of figure = 900 cm2

10. Top surface of a table is trapezium in shape. Find its area if its parallel sides are 1 m and 1.2 m and perpendicular distance between them is 0.8 m.

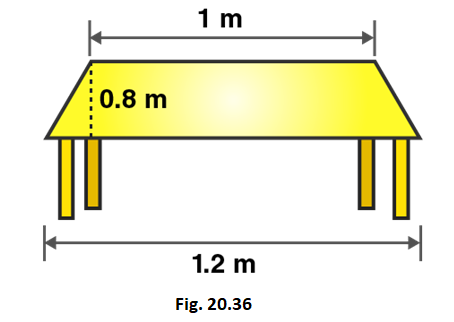
Solution:

Given that,

Length of parallel sides of trapezium = 1.2m and 1m

Distance between parallel sides of trapezium = 0.8m

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

Area of trapezium = 1/2 (1.2 + 1) × 0.8

Area of trapezium = 1/2 × 2.2 × 0.8 = 0.88

So, Area of trapezium = 0.88m2

11. The cross-section of a canal is a trapezium in shape. If the canal is 10 m wide at the top 6 m wide at the bottom and the area of cross-section is 72 m2 determine its depth.

Solution:

Given that,

Length of parallel sides of trapezium = 10m and 6m

Area = 72 m2 

Let the distance between parallel sides of trapezium = x meter

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

72 = 1/2 (10 + 6) × x

72 = 8 × x

x = 72/8 = 9

∴ The depth is 9m.

12. The area of a trapezium is 91 cm2 and its height is 7 cm. If one of the parallel sides is longer than the other by 8 cm, find the two parallel sides.

Solution:

Given that,

Let the length of one parallel side of trapezium = x meter

Length of other parallel side of trapezium = (x+8) meter

Area of trapezium = 91 cm2

Height = 7 cm

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

91 = 1/2 (x+x+8) × 7

91 = 1/2(2x+8) × 7

91 = (x+4) × 7

(x+4) = 91/7

x+4 = 13

x = 13 – 4

x = 9

∴ Length of one parallel side of trapezium = 9 cm

And, Length of other parallel side of trapezium = x+8 = 9+8 = 17 cm

13. The area of a trapezium is 384 cm2. Its parallel sides are in the ratio 3:5 and the perpendicular distance between them is 12 cm. Find the length of each one of the parallel sides.

Solution:

Given that,

Let the length of one parallel side of trapezium = 3x meter

Length of other parallel side of trapezium = 5x meter

Area of trapezium = 384 cm2

Distance between parallel sides = 12 cm

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

384 = 1/2 (3x + 5x) × 12

384 = 1/2 (8x) × 12

4x = 384/12

4x = 32

x = 8

∴ Length of one parallel side of trapezium = 3x = 3× 8 = 24 cm

And, Length of other parallel side of trapezium = 5x = 5× 8 = 40 cm

14. Mohan wants to buy a trapezium shaped field. Its side along the river is parallel and twice the side along the road. If the area of this field is 10500 m2 and the perpendicular distance between the two parallel sides is 100 m, find the length of the side along the river.

Solution:

Given that,

Let the length of side of trapezium shaped field along road = x meter

Length of other side of trapezium shaped field along road = 2x meter

Area of trapezium = 10500 cm2

Distance between parallel sides = 100 m

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

10500 = 1/2 (x + 2x) × 100

10500 = 1/2 (3x) × 100

3x = 10500/50

3x = 210

x = 210/3 = 70

x = 70

∴ Length of side of trapezium shaped field along road = 70 m

And, Length of other side of trapezium shaped field along road = 2x = 70× 2 = 140 m

15. The area of a trapezium is 1586 cm2 and the distance between the parallel sides is 26 cm. If one of the parallel sides is 38 cm, find the other.

Solution:

Given that,

Let the length of other parallel side of trapezium = x cm

Length of one parallel side of trapezium = 38 cm

Area of trapezium = 1586 cm2

Distance between parallel sides = 26 cm

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

1586 = 1/2 (x + 38) × 26

1586 = (x + 38) × 13

(x + 38) = 1586/13

x = 122 – 38

x =84

∴ Length of the other parallel side of trapezium = 84 cm

16. The parallel sides of a trapezium are 25 cm and 13 cm; its nonparallel sides are equal, each being 10 cm, find the area of the trapezium.

Solution:

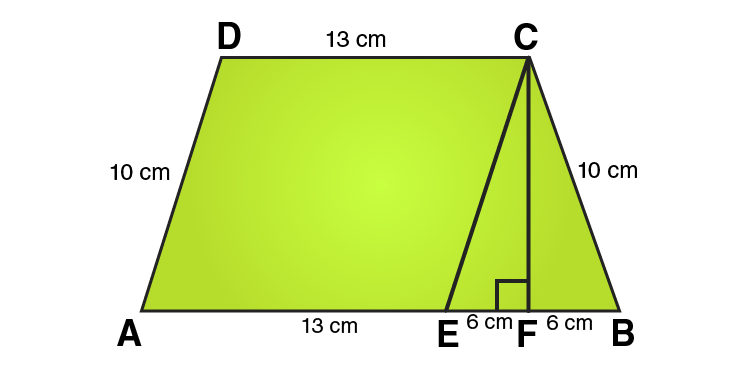
In ΔCEF,

CE = 10 cm and EF = 6cm

Using Pythagoras theorem:

CE2 = CF2 + EF2

CF2 = CE2 – EF2

CF2 = 102 – 62

CF2 = 100-36

CF2 = 64

CF = 8 cm

From the figure we can write,

Area of trapezium = Area of parallelogram AECD + Area of area of triangle CEF

Area of trapezium = base × height + 1/2 (base × height)

Area of trapezium = 13 × 8 + 1/2 (12 × 8)

Area of trapezium = 104 + 48 = 152

∴ Area of trapezium = 152 cm2

17. Find the area of a trapezium whose parallel sides are 25 cm, 13 cm and the other sides are 15 cm each.

Solution:

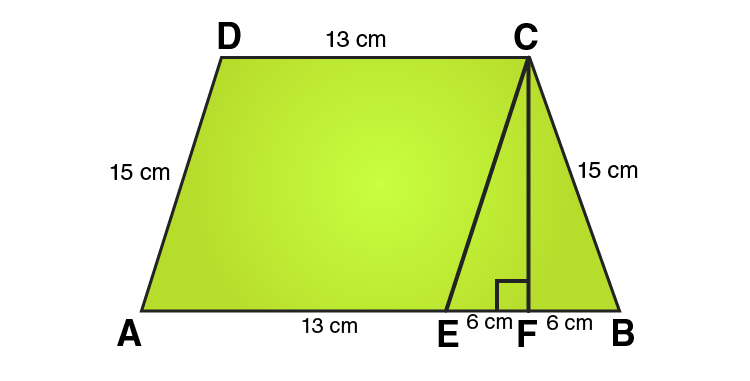
In ΔCEF,

CE = 10 cm and EF = 6cm

Using Pythagoras theorem:

CE2 = CF2 + EF2

CF2 = CE2 – EF2

CF2 = 152 – 62

CF2 = 225-36

CF2 = 189

CF = √189

= √ (9×21)

= 3√21 cm

From the figure we can write,

Area of trapezium = Area of parallelogram AECD + Area of area of triangle CEF

Area of trapezium = height + 1/2 (sum of parallel sides)

Area of trapezium = 3√21 × 1/2 (25 + 13)

Area of trapezium = 3√21 × 19 = 57√21

∴ Area of trapezium = 57√21 cm2

18. If the area of a trapezium is 28 cm2 and one of its parallel sides is 6 cm, find the other parallel side if its altitude is 4 cm.

Solution:

Given that,

Let the length of other parallel side of trapezium = x cm

Length of one parallel side of trapezium = 6 cm

Area of trapezium = 28 cm2

Length of altitude of trapezium = 4 cm

We know that,

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × distance between parallel sides

i.e., Area of trapezium = 1/2 (Sum of sides) × distance between parallel sides

28 = 1/2 (6 + x) × 4

28 = (6 + x) × 2

(6 + x) = 28/2

(6 + x) = 14

x = 14 – 6

x = 8

∴ Length of the other parallel side of trapezium = 8 cm

19. In Fig. 20.38, a parallelogram is drawn in a trapezium, the area of the parallelogram is 80 cm2, find the area of the trapezium.

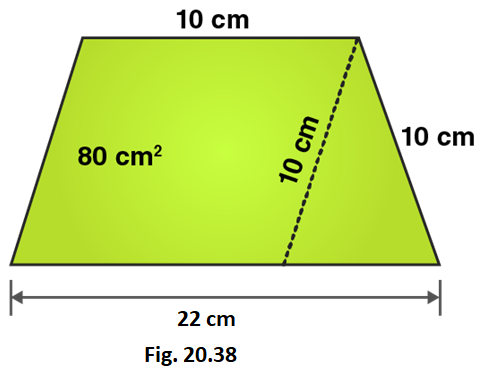
Solution:

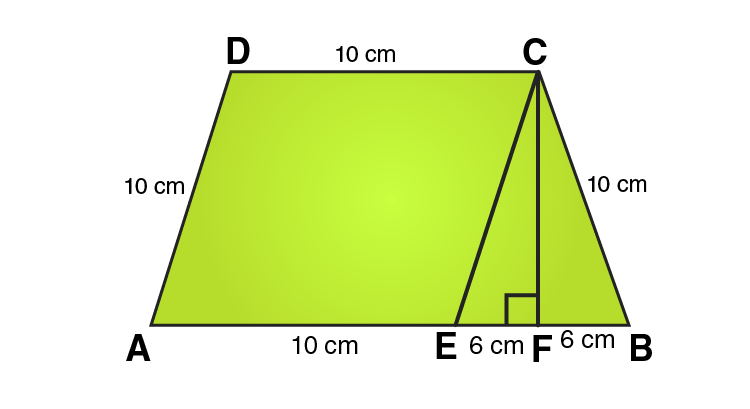
In ΔCEF,

CE = 10 cm and EF = 6cm

Using Pythagoras theorem:

CE2 = CF2 + EF2

CF2 = CE2 – EF2

CF2 = 102 – 62

CF2 = 100-36

CF2 = 64

CF = 8 cm

Area of parallelogram = 80 cm2

From the figure we can write,

Area of trapezium = Area of parallelogram AECD + Area of area of triangle CEF

Area of trapezium = base × height + 1/2 (base × height)

Area of trapezium = 10 × 8 + 1/2 (12 × 8)

Area of trapezium = 80 + 48 = 128

∴ Area of trapezium = 128 cm2

20. Find the area of the field shown in Fig. 20.39 by dividing it into a square, a rectangle and a trapezium.

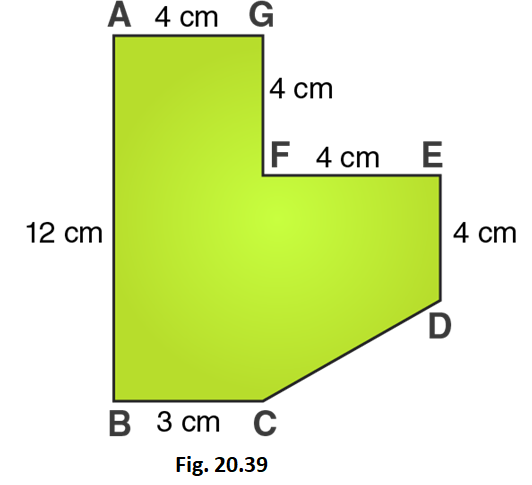
Solution:

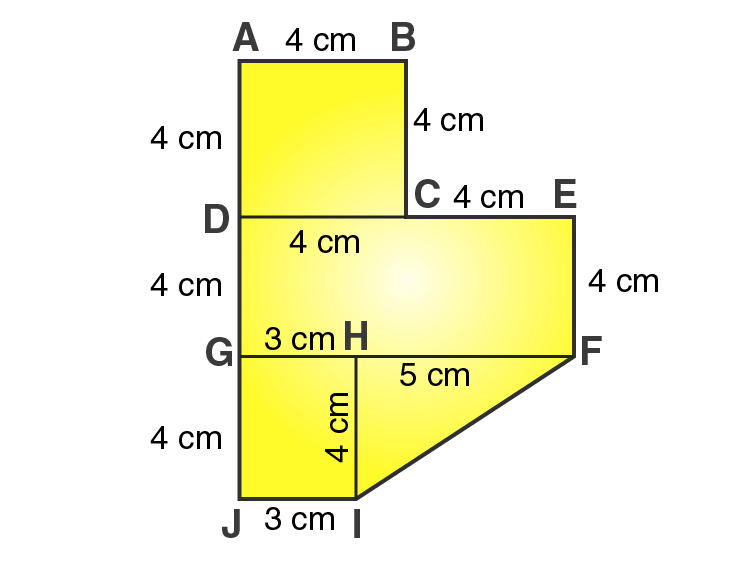
From the figure we can write,

Area of given figure = Area of square ABCD + Area of rectangle DEFG + Area of rectangle GHIJ + Area of triangle FHI

i.e., Area of given figure = side × side + length × breadth + length × breadth + 1/2 × base × altitude

Area of given figure = 4×4 + 8×4 + 3×4 + 1/2×5×5

Area of given figure = 16 + 32 + 12 + 10 = 70

∴ Area of given figure = 70 cm2

---

EXERCISE 20.3 PAGE NO: 20.28

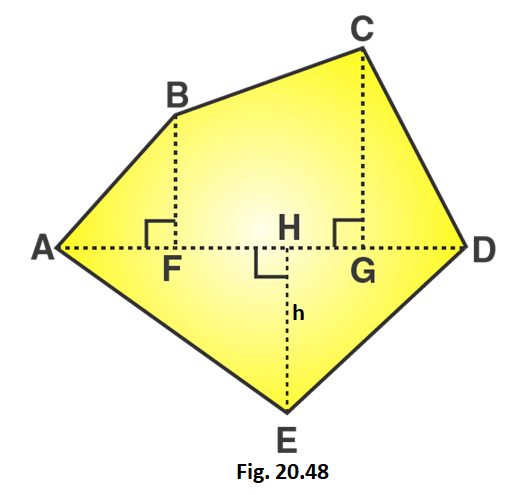
1. Find the area of the pentagon shown in fig. 20.48, if AD = 10 cm, AG = 8 cm, AH = 6 cm, AF = 5 cm, BF = 5 cm, CG = 7 cm and EH = 3 cm.

Solution:

GH = AG – AH = 8 – 6 = 2 cm

HF = AH – AF = 6 – 5 = 1 cm

GD = AD – AG = 10 – 8 = 2 cm

From the figure we can write,

Area of given figure = Area of triangle AFB + Area of trapezium BCGF + Area of triangle CGD + Area of triangle AHE + Area of triangle EGD

We know that,

Area of right angled triangle = 1/2 × base × altitude

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of given pentagon = 1/2 × AF × BF + 1/2 (CG + BF) × FG + 1/2 × GD × CG + 1/2 × AH × EH + 1/2 × HD × EH

Area of given pentagon = 1/2 × 5 × 5 + 1/2 (7 + 5) × 3 + 1/2 × 2 × 7 + 1/2 × 6 × 3 + 1/2 × 4 × 3

Area of given pentagon = 12.5 + 18 + 7 + 9 + 6 = 52.5

∴ Area of given pentagon = 52.5 cm2

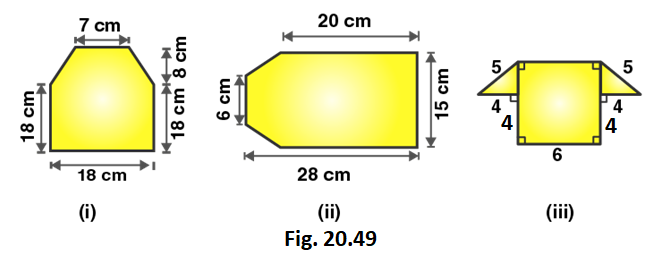
2. Find the area enclosed by each of the following figures [fig. 20.49 (i)-(ii)] as the sum of the areas of a rectangle and a trapezium.

Solution:

Figure (i)

From the figure we can write,

Area of figure = Area of trapezium + Area of rectangle

Area of figure = 1/2 (Sum of lengths of parallel sides) × altitude + Length × Breadth

Area of figure = 1/2 (18 + 7) × 8 + 18 × 18

Area of figure = 1/2 (25) × 8 + 18 × 18

Area of figure =

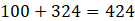
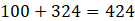
∴ Area of figure is 424 cm2

Figure (ii)

From the figure we can write,

Area of figure = Area of trapezium + Area of rectangle

Area of figure = 1/2 (Sum of lengths of parallel sides) × altitude + Length × Breadth

Area of given figure = 1/2 (15 + 6) × 8 + 15 × 20

Area of given figure = 84 + 300 = 384

∴ Area of figure is 384 cm2

Figure (iii)

Using Pythagoras theorem in the right angled triangle,

52 = 42 + x2

x2 = 25 – 16

x2 = 9

x = 3 cm

From the figure we can write,

Area of figure = Area of trapezium + Area of rectangle

Area of figure = 1/2 (Sum of lengths of parallel sides) × altitude + Length × Breadth

Area of given figure = 1/2 (14 + 6) × 3 + 4 × 6

Area of given figure = 30 + 24 = 54

∴ Area of figure is 54 cm2

3. There is a pentagonal shaped park as shown in Fig. 20.50. Jyoti and Kavita divided it in two different ways.
Find the area of this park using both ways. Can you suggest some another way of finding its area?

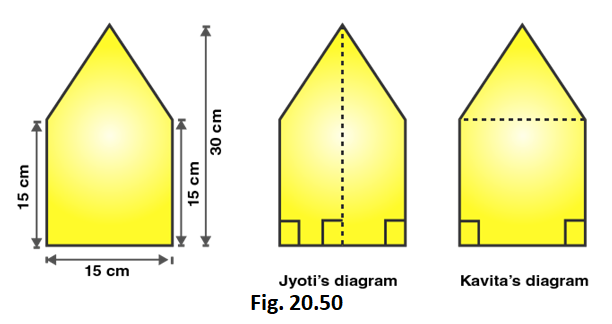
Solution:

From the figure we can write,

Area of figure = Area of trapezium + Area of rectangle

Area of Jyoti’s diagram = 2 × 1/2 (Sum of lengths of parallel sides) × altitude

Area of figure = 2 × 1/2 × (15 + 30) × 7.5

Area of figure = 45 × 7.5 = 337.5

Therefore, Area of figure = 337.5 cm2

We also know that,

Area of Pentagon = Area of triangle + area of rectangle

Area of Pentagon = 1/2 × Base × Altitude + Length × Breadth

Area of Pentagon = 1/2 × 15 × 15 + 15 × 15

Area of Pentagon = 112.5 + 225 = 337.5

∴ Area of pentagon is 337.5 m2

4. Find the area of the following polygon, if AL = 10 cm, AM = 20 cm, AN = 50 cm. AO = 60 cm and AD = 90 cm.

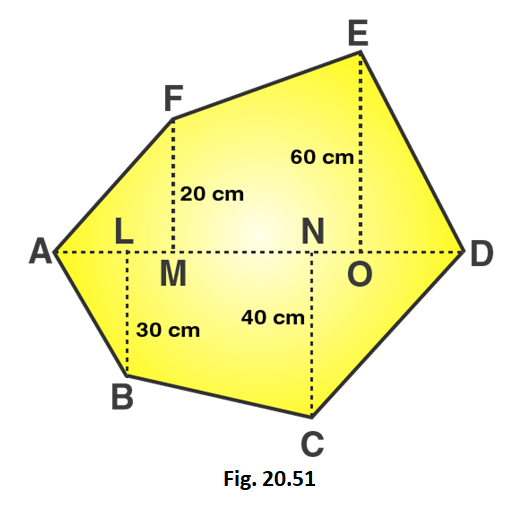
Solution:

Given that,

AL = 10 cm; AM = 20 cm; AN = 50 cm; AO = 60 cm; AD = 90 cm

LM = AM – AL = 20 – 10 = 10 cm

MN = AN – AM = 50 – 20 = 30 cm

OD = AD – AO = 90 – 60 = 30 cm

ON = AO – AN = 60 – 50 = 10 cm

DN = OD + ON = 30 + 10 = 40 cm

OM = MN + ON = 30 + 10 = 40 cm

LN = LM + MN = 10 + 30 = 40 cm

From the figure we can write,

Area of figure = Area of triangle AMF + Area of trapezium FMNE + Area of triangle END + Area of triangle ALB + Area of trapezium LBCN + Area of triangle DNC

We know that,

Area of right angled triangle = 1/2 × base × altitude

Area of trapezium = 1/2 (Sum of lengths of parallel sides) × altitude

Area of given hexagon = 1/2 × AM × FM + 1/2 (MF + OE) × OM + 1/2 × OD × OE + 1/2 × AL × BL + 1/2 × (BL + CN) × LN + 1/2 × DN × CN

Area of given hexagon = 1/2 × 20 × 20 + 1/2 (20 + 60) × 40 + 1/2 × 30 × 60 + 1/2 × 10 × 30 + 1/2 × (30 + 40) × 40 + 1/2 × 40 × 40

Area of given hexagon = 200 + 1600 + 900 + 150 + 1400 + 800 = 5050

∴ Area of given hexagon is 5050 cm2

5. Find the area of the following regular hexagon.

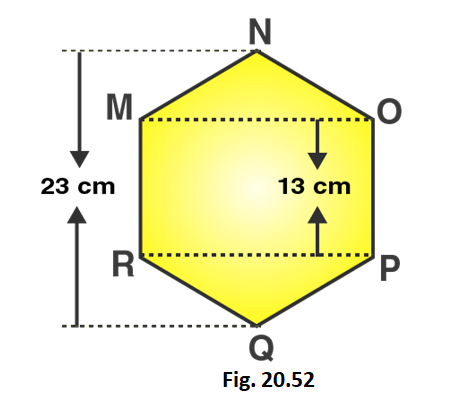
Solution:

Given that,

NQ = 23 cm

ND + QC = 23 -13 = 10

ND = QC = 5 cm

OM = 24 cm

Area of given hexagon = 2 times Area of triangle MNO + Area of rectangle MOPR

Area of right angled triangle = 1/2 × base × altitude

Area of rectangle = length × breadth

Area of regular hexagon = 1/2 × OM × ND + MO × OP + 1/2 × RP × QC

Area of regular hexagon = 1/2 × 24 × 5 + 24 × 13 + 1/2 × 24 × 5

Area of given hexagon = 1/2 × 120 + 312 + 1/2 × 120 = 432

∴ Area of given hexagon is 432 cm2